The multiplicative sum Zagreb index is a modified version of the well-known Zagreb indices. The multiplicative sum Zagreb index of a graph
In this paper, we focus exclusively on undirected simple connected
graphs. Let
Topological indices are numbers that reflect certain structural
features of organic molecules derived from the molecular graph. They
play an important role in chemistry, pharmacology, etc. (see [1-3]). One
of the oldest topological indices is the well-known Zagreb index, first
introduced in [4],
where Gutman and Trinajsti
These two classical topological indices (
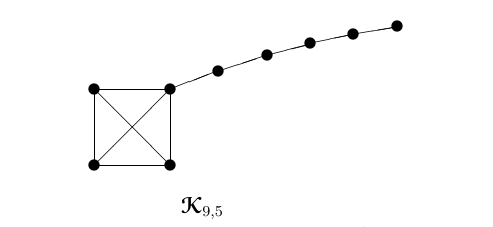
Let
In recent years, many scholars have been interested in finding the
extremal values of topological indices with a given clique number [20-25].
Hence, by using graph transformations and properties of the
multiplicative sum Zagreb index, we show here that for the graphs in
By the definition of the multiplicative sum Zagreb index, the following Lemma 2.1 is obvious and can be found in [6].
Lemma 1. [6] Let
If
If
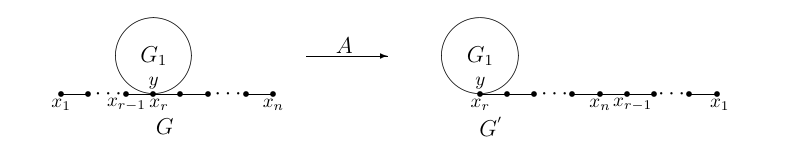
Definition 1 (Transformation
Lemma 2. [6] Let
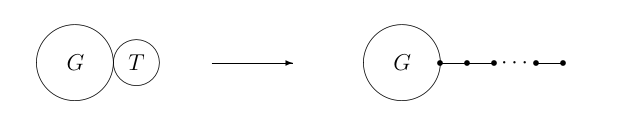
Corollary 1. [6] By repeating Transformation
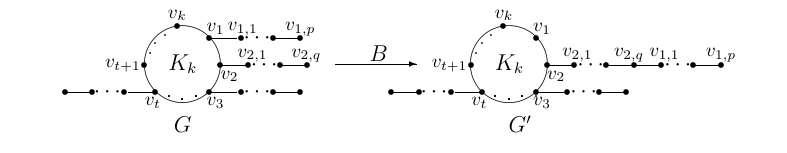
Definition 2 (Transformation
Lemma 3. Let
Proof. We notice that
If
If
If
If
When
Let
Theorem 1. Let
Proof. Choose a graph
If
By the definition of multiplicative sum Zagreb index, we have
Let
Lemma 4. Let
Proof. By the definition of the set
In order to get the maximum multiplicative sum Zagreb index, we first
consider the multiplicative sum Zagreb indices of graphs
Theorem 2. Let
Proof. In view of the definition of chromatic number, any
graph
Now we claim that
By the definition of the multiplicative sum Zagreb index, we have
Recall that
Conversely, it is easy to see that the equality holds when
Lemma 5. [26] Let
Theorem 3. Let
Proof. Choose a graph
By Theorem 2, considering the uniqueness of the extremal
graph in the set
Recently, the extremal values of the multiplicative Zagreb-related
indices of graphs with given graph parameters have been widely explored.
At present, we have obtained the maximum and minimum multiplicative sum
Zagreb indices on the graphs of order
Sun is supported by the Natural Science Foundation of Shanxi Province of China (202303021211154).
Gutman, I. and Furtula (Eds.) B., 2010. Novel Molecular Structure Descriptors – Theory and Applications I. University of Kragujevac, Kragujevac.
Gutman, I. and Furtula (Eds.) B., 2010. Novel Molecular Structure Descriptors – Theory and Applications II. University of Kragujevac, Kragujevac.
Todeschini, R. and Consonni, V., 2000. Handbook of Molecular Descriptors. Wiley-VCH, Weinheim.
Gutman, I. and Trinajsti
Eliasi, M., Iranmanesh, A. and Gutman, I., 2012. Multiplicative versions of first Zagreb index. MATCH Communications in Mathematical and in Computer Chemistry, 68, pp.217-230.
Xu, K. and Das, K. Ch., 2012. Trees, unicyclic, and bicyclic graphs extremal with respect to multiplicative sum Zagreb index. MATCH Communications in Mathematical and in Computer Chemistry, 68, pp.257-272.
Bo
Azari, M. and Iranmanesh, A., 2015. Some inequalities for the multiplicative sum Zagreb index of graph operations. Journal of Mathematical Inequalities, 9, pp.727-738.
Du, J. and Sun, X., 2020. On the multiplicative sum Zagreb index of graphs with some given parameters. Journal of Mathematical Inequalities, 14, pp.1165-1181.
Sun, X., Gao, Y. and Du, J., 2023. On multiplicative sum Zagreb index of trees with fixed domination number. Journal of Mathematical Inequalities, 17, pp.83-98.
Ali, A., Noureen, S., Moeed, A., Iqbal, N. and Hassan, T. S., 2024. Fixed-order chemical trees with given segments and their maximum multiplicative sum Zagreb index. Mathematics, 12, p.1259.
Dehgardi, N., Du, Z. and Shang, Y., 2024. Multiplicative Sombor index of trees. Notes on Number Theory and Discrete Mathematics, 30, pp.453-460.
Du, J. and Sun, X., 2023. Extremal quasi-unicyclic graphs with respect to the general multiplicative Zagreb indices. Discrete Applied Mathematics, 325, pp.200-211.
Du, J. and Sun, X., 2024. On bond incident degree index of chemical trees with a fixed order and a fixed number of leaves. Applied Mathematics and Computation, 464, p.128390.
Horoldagva, B., Xu, C., Buyantogtokh, L. and Dorjsembe, S., 2020. Extremal graphs with respect to the multiplicative sum Zagreb index. Match Communications in Mathematical and in Computer Chemistry, 84, pp.773-786.
Ismail, R., Azeem, M., Shang, Y., Imran, M. and Ahmad, A., 2023. A unified approach for extremal general exponential multiplicative Zagreb indices. Axioms, 12, p.675.
Noureen, S., Ali, A., Bhatti, A. A., Alanazi, A. M. and Shang, Y., 2024. Predicting enthalpy of formation of benzenoid hydrocarbons and ordering molecular trees using general multiplicative Zagreb indices. Heliyon, 10, p.e30913.
Xu, C., Horoldagva, B. and Buyantogtokh, L., 2021. Cactus graphs with maximal multiplicative sum Zagreb index. Symmetry, 13, p.913.
Bondy, J. A. and Murty, U. S. R., 1976. Graph Theory with Applications. Elsevier, New York.
Du, J., Shao, Y. and Sun, X., 2020. The zeroth-order general Randić index of graphs with a given clique number. Korean Journal of Mathematics, 28, pp.405-419.
Vetrík, T. and Balachandran, S., 2019. General multiplicative Zagreb indices of graphs with given clique number. Opuscula Mathematics, 39, pp.433-446.
Wali, M. and Guji, R., 2024. Extremal Sombor index of graphs with cut edges and clique number. Axioms, 13, p.66.
Xu, K., 2011. The Zagreb indices of graphs with a given clique number. Applied Mathematics Letters, 24, pp.1026-1030.
Xu, K., 2010. On the Hosoya index and the Merrifield-Simmons index of graphs with a given clique number. Applied Mathematics Letters, 23, pp.395-398.
Yang, J., Liu, H. and Wang, Y., 2024. Vertex-degree function index for concave functions of graphs with a given clique number. Journal of Applied Mathematics and Computing, 70, pp.2197-2208.
Erd
1970-2025 CP (Manitoba, Canada) unless otherwise stated.