On Some Topological Indices for the Orbit Graph of Dihedral Groups

Bhat, Vijay; Sharma, Karnika

doi:/10.61091/jcmcc117-18

Abstract

1. Introduction
2. Preliminaries
3. Indices for orbit graph of Dt
4. Relation with wiener index
5. Polynomial for the Indices of Dt
6. Conclusion
Acknowledgement

References

Journal of Combinatorial Mathematics and Combinatorial Computing

Volume 117
Pages: 195-208

Research article

On Some Topological Indices for the Orbit Graph of Dihedral Groups

^¹, ^¹

¹School of Mathematics, Shri Mata Vaishno Devi University, Katra-182320, Jammu and Kashmir, India.

Received: 28/11/2023
Accepted: 02/12/2023
Published: 11/12/2023

Copyright Link
License

Abstract

Let $Γ_{G}$ be the orbit graph of $G$ , with non-central orbits in the subset of order two commuting elements in $G$ , and the vertices of $Γ_{G}$ connected if they are conjugate. The main objective of this study is to compute several topological indices for the orbit graph of a dihedral group, including the Wiener index, the Zagreb index, the Schultz index, and others. We also develop a relationship between the Wiener index and the other indices for the dihedral group’s orbit graph. Furthermore, their polynomial has been computed as well.

Keywords: Wiener Index, Zagreb Index, Schultz Index, Somber Index, Randic Index, Dihedral group, Orbit graph

1. Introduction

Graph theory has provided us with several useful tools, one of the most studied and applicable tools is the topological index. Topological indices (TIs) are the numerical parameters of a graph that can be employed to characterize its topology. A topological index is also known as a connectedness index in chemical graph theory, molecular topology, and mathematical chemistry domains. A molecule can be represented as a graph in the chemical graph theory, with atoms as vertices and bonds as edges. Topological graph indices have been successfully utilized to predict specific physicochemical properties as well as to determine the structural properties of chemical compounds. Stankevich et al. [1] examined topological indices as one way of identifying the relationship between the structure of the chemical compound and its characteristics. Topological indices have also assisted chemists, physicians, mathematicians, and others in solving chemical and biological problems.
Diudea et al. [2] proposed a unified approach to the Wiener topological index and its various modifications, where they focus on the Schultz, Cluj, Szeged, Harary, and Kirchhoff indices, as well as their numerous variants and generalizations. They also obtained the relationship between these indices and their correlations with the physicochemical properties of molecules. Cancan et al. [3] employed the findings obtained in [2] to investigate the generalized prism network. Further, they have computed several degree-based indices such as the Airthmetic-Geometric index, modified Randic, sum connectivity index, the SK index, $S K_{1}$ index, and $S K_{2}$ index of generalised prism network engineering.
The numerical representations of chemical structures with topological indices are significant in medicinal chemistry and bioinformatics. In the early 1990s, the invention and specification of the new TIs increased consistently. Topological indices include degree-based, distance-based, counting-related, and many other topological indices. These indices are used to create quantitative structure-activity relationships, in which a molecule’s biological activity, as well as other properties like strain energy, stability, and boiling point, are linked to its structure. To classify these indices, the structural properties of the graphs are used to calculate the TIs. For example, Zagreb indices are derived using the degrees of vertices and the wiener index is obtained by using the distance between of vertices in the given molecular graph. In 1947, [4] the first graph-based molecular structure TI was discovered. Wiener has provided only two important topological indices; the Wiener Index $W (G)$ , and the Wiener polarity index $W_{p}$ . These indices are used to obtain the chemical and physical properties of chemical compounds. Some formulas comprising terms of this kind were deduced a long time ago while studying the dependence of total $π$ -electron energy on molecule structure. That is, $\begin{array}{rcl} M_{1} = & \sum_{v e r t i c e s} (d_{i})^{2} \\ M_{2} = & \sum_{e d g e s} d_{i} . d_{j} \end{array}$ with $d_{i}$ standing for the degree of the vertex $v_{i}$ of the molecular graph [4]. In the chemical literature, $M_{1}$ and $M_{2}$ are called the first Zagreb index and the second Zagreb index respectively.
Many studies have been reported regarding the applications of topological indices in the last four decades. Das et al. [5] introduced several significant characteristics of the second Zagreb index. In their work, they have declared and verified numerous findings for $M_{2}$ identities and inequalities. The M-polynomial for the graph has received a lot of attention since it generates a lot of degree-based topological indices. Munir et al. [6] used this method to determine M-polynomials of various nanostar dendrimers and subsequently retrieve a large number of degree-based topological indices. Zhou et al. [7] derive several inequalities of three previously investigated descriptors, namely the Zagreb, Wiener, and Hyper-Wiener indices concerning the given molecular graph. Khalifeh et al. [8] gave some exact formulae for the first and second Zagreb indices of graph operations containing the symmetric difference, composition, disjunction, join, and cartesian product of graphs. For the nanostructure of bridge graphs, Khalaf et al. [9] determine the hyper-Zagreb index, first multiple Zagreb index, second multiple index, Zagreb polynomials, and M-polynomials. Das et al. [10] compared the Wiener index and the Zagreb indices, as well as the eccentric connectivity index for trees. Bello et al. [11] generalized the Wiener index, first and second Zagreb indices of the ordered product prime graph on dihedral groups. Singh and Bhat [12] investigated the adjacency matrix and various topological indices of the zero-divisor graph of $Z_{n}$ . Shahistha et al. [13] calculate the Wiener index of some significant chain graphs.
In the recent past, Poojary et al. [14] developed several topological indices and polynomials for the Issac graph. Filipovski [15] established several relationships between the Sombor index and degree-based topological indices such as the Zagreb index, Forgotten index, and Randic index. Saleh et al. [16] introduced the first, second, and forgotten downhill Zagreb indices of graphs. Saeed et al. [17] investigated degree-based topological indices of Boron $B_{12}$ , such as the Randic index, the first general Zagreb index, the hyper-Zagreb index, and others. Javaid et al. [18] calculated novel connection-based Zagreb indices for many wheel-related graphs such as the wheel, gear, helm, flower, and sunflower graphs. Because of their uses, entire versions of numerous indices have been introduced and investigated. Naci et al. [19] presented the Wiener index for trees and some graph families.
The above-mentioned applications motivate us to study topological indices for the orbit graph of $G$ . This study examines the Wiener index, the first, second, and third Zagreb indices, Hyper first and second Zagreb indices, Modified first Zagreb indices, Schultz and Modified Schultz indices, the Forgotten index, Somber index, Randic and Reciprocal Randic indices for the orbit graph of the dihedral group $D_{t}$ . Furthermore, we also obtain the relationships between these indices with the wiener index and the polynomials of indices for the orbit graphs of $D_{t}$ .

2. Preliminaries

The following definitions related to the group theory, graph theory and topological indices are stated as below.

Definition 1. Dihedral Group: Let $G$ be a dihedral group of order $2 t$ and is represented as
$D_{t} = ⟨ a, b | a^{t} = b^{2} = e, b a b = a^{- 1} ⟩$ with $t$ being a positive integer and $t \geq 3$ .

Definition 2. [20]The Set $Υ$ : The set $Υ$ is the set of all pairs of commuting elements of $G$ and the least common multiple of the elements having order two and is represented as
$Υ = {(a_{1}, b_{1}) \in G \times G | a_{1} b_{1} = b_{1} a_{1}, a_{1} \neq b_{1}, l c m (| a_{1} |, | b_{1} |) = 2} .$

Definition 3. [20]Orbit: If a group $G$ operates on a set $Υ$ and $v_{1} \in Υ$ , then the subset $R (v_{1}) = {g v_{1} ∣ g \in G, v_{1} \in Υ}$ is the orbit of $v_{1}$ and is symbolized by $R (v_{1})$ . In this study, the conjugation action is considered. Therefore, the orbit is written as
$R (v_{1}) = {g v_{1} g^{- 1} ∣ g \in G, v_{1} \in Υ} .$

Definition 4. [20]Orbit Graph $Γ_{G}^{Υ}$ : The orbit graph, $Γ_{G}^{Υ}$ is a graph whose vertices are non central orbits under group action on the set $Υ$ that is $| V (Γ_{G}^{Υ}) | = | Υ | - | B |$ , where $Υ$ is a disjoint union of distinct orbits and $B = {v_{1} \in Υ | v_{1} g = g v_{1}, g \in G}$ . Two vertices $v_{1}, v_{2}$ are adjacent if $v_{1}, v_{2}$ are conjugate that is $v_{1} = g^{v_{2}}$ .

The following are some definitions that are used in computing the topological indices.

Definition 5. [21]Degree of a vertex: The number of incident edges $| E (u) |$ on the vertex $u$ is called degree of a vertex and is denoted by $d e g (u)$ .

Definition 6. [21]Distance: The minimum number of edges between two vertices $u_{i}$ and $u_{j}$ of a graph is said to be a distance of two vertices and is denoted by $d (u_{i}, u_{j})$ , where $i$ , $j$ denotes the number of vertices.

Let $u_{i}$ and $u_{j}$ be two unique vertices with $i \leq j$ and $Γ_{G}^{Υ}$ a connected graph of $t$ vertices, respectively. Then there are the definitions of several indices that we utilise in this work, which are listed in Table $1$ .

Topological Indices Formulas
Indices	Formulas
$W (Γ_{G}^{Υ})$	$\frac{1}{2} \sum_{i = 1}^{t} \sum_{j = 1}^{t} d (u_{i}, u_{j})$
$M_{1} (Γ_{G}^{Υ})$	$\sum_{u \in v (Γ_{G}^{Υ})} (d e g (u))^{2}$
$M_{2} (Γ_{G}^{Υ})$	$\sum_{u_{1}, u_{2} \in E (Γ_{G}^{Υ})} d e g (u_{1}) d e g (u_{2})$
$M_{3} (Γ_{G}^{Υ})$	$\sum_{i, j = 1}^{\frac{t (t - 1)}{2}} (d e g (u_{i}) d e g (u_{j})) (d e g (u_{i}) + d e g (u_{j}))$
$H M_{1} (Γ_{G}^{Υ})$	$\sum_{i, j = 1}^{\frac{t (t - 1)}{2}} (d e g (u_{i}) + d e g (u_{j}))^{2}$
$H M_{2} (Γ_{G}^{Υ})$	$\sum_{i, j = 1}^{\frac{t (t - 1)}{2}} (d e g (u_{i}) d e g (u_{j}))^{2}$
$M o d M_{1} (Γ_{G}^{Υ})$	$\sum_{i, j = 1}^{t} \frac{1}{d e g (u_{i})^{2}}$
$S_{c} (Γ_{G}^{Υ})$	$\frac{1}{2} \sum (d e g (u_{i}) + d e g (u_{j})) d (u_{i}, u_{j})$
$S_{c}^{*} (Γ_{G}^{Υ})$	$\frac{1}{2} \sum (d e g (u_{i}) d e g (u_{j})) d (u_{i}, u_{j})$
$F (Γ_{G}^{Υ})$	$\sum_{i, j = 1}^{\frac{t (t - 1)}{2}} d e g (u_{i})^{2} + d e g (u_{j})^{2}$
$S_{o} (Γ_{G}^{Υ})$	$\sum_{i, j = 1}^{\frac{t (t - 1)}{2}} (d e g (u_{i})^{2} + d e g (u_{j})^{2})^{\frac{1}{2}}$
$R (Γ_{G}^{Υ})$	$\sum_{i, j = 1}^{\frac{t (t - 1)}{2}} (d e g (u_{i}) d e g (u_{j})) \frac{- 1}{2}$
$R R (Γ_{G}^{Υ})$	$\sum_{i, j = 1}^{\frac{t (t - 1)}{2}} (d e g (u_{i}) d e g (u_{j}))^{\frac{1}{2}}$

The orbit graph of all dihedral groups, $D_{t}$ , can be divided into three parts, according to Omer et al., [20], as stated in the following theorem. when $t$ and $\frac{t}{2}$ are even, $| Υ | = \frac{5 t}{2} + 1$ and $| B | = 1$ , when $t$ is even and $\frac{t}{2}$ is odd, $| Υ | = \frac{5 t}{2} + 1$ and $| B | = 1$ and when $t$ is odd, $| Υ | = t$ and $| B | = 0$ . This fact will be utilised to support our primary findings.

Theorem 1. [20]Let $G$ be a dihedral group of order $2 t$ . If $G$ acts on $Υ$ by conjugation. Then $Γ_{G}^{Υ} = {\begin{cases} ⋃_{i = 1}^{5} K_{\frac{t}{2} i}, & if t is even and \frac{t}{2} is odd, \\ (⋃_{i = 1}^{4} K_{\frac{t}{2} i}) ⋃ (⋃_{i = 1}^{2} K_{\frac{t}{4}} i), & if t and \frac{t}{2} is odd, \\ K_{t}, & if t is odd . \end{cases}$

3. Indices for orbit graph of $D_{t}$

According to Theorem 1, only the third case involves a connected graph, while the other two cases involve disconnected graphs. As a result, we can only think about the case where $t$ is odd. In this section, the Wiener index, the first, second and third Zagreb indices, Hyper first and second Zagreb indices, Modified first Zagreb indices, Schultz and Modified Schultz indices, Forgotten index, Somber index, Randic and Reciprocal Randic indices for the orbit graph of dihedral groups are calculated.

Theorem 2. Let $G$ be a dihedral group $D_{t}$ of order $2 t$ . Then

$W (Γ_{G}^{Υ})$ = $\frac{1}{2} t (t - 1)$
$M_{1} (Γ_{G}^{Υ}$ = $t (t - 1)^{2}$
$M_{2} (Γ_{G}^{Υ})$ = $\frac{t (t - 1)^{3}}{2}$
$M_{3} (Γ_{G}^{Υ})$ = $t (t - 1)^{4}$
$H M_{1} (Γ_{G}^{Υ})$ = $2 t (t - 1)^{3}$
$H M_{2} (Γ_{G}^{Υ})$ = $\frac{t (t - 1)^{5}}{2}$
$M o d M_{1} (Γ_{G}^{Υ})$ = $\frac{t}{(t - 1)^{2}}$
$S_{c} (Γ_{G}^{Υ})$ = $t (t - 1)^{2}$
$S_{c}^{*} (Γ_{G}^{Υ})$ = $\frac{t (t - 1)^{3}}{2}$
$F (Γ_{G}^{Υ})$ = $t (t - 1)^{3}$
$S_{o} (Γ_{G}^{Υ})$ = $t (t - 1)^{2}$
$R (Γ_{G}^{Υ})$ = $\frac{t}{2}$
$R R (Γ_{G}^{Υ})$ = $\frac{t (t - 1)^{2}}{2}$

Proof. In order to calculate the indices of a graph, we have to determine the number of vertices of the graph. From the definition $2.4$ , if the group action on the set $Υ$ is applied then $| Υ | - | B | = t - 0 = t$ and the number of vertices of the orbit graph is considered to be a non-central orbit.
Since, we are considering the third case of the Theorem 1, where the graph is complete then the number of edges of the orbit graph are $\frac{t (t - 1)}{2}$ and distance and degree of the vertex is $(t - 1)$ (All the indices are determined on the basis of above predefined definitions). Therefore, $\begin{array}{rcl} W (Γ_{G}^{Υ}) & = & \frac{1}{2} \sum_{i = 1}^{t} \sum_{j = 1}^{t} d (u_{i}, u_{j}) \\ = & \frac{1}{2} \sum_{i = 1}^{t} [d (u_{i}, u_{1}) + d (u_{i}, u_{2}) + \dots + d (u_{i}, u_{t})] \\ = & \frac{1}{2} [d (u_{1}, u_{1}) + d (u_{2}, u_{1}) + \dots + d (u_{t}, u_{1}) + \dots + \\ d (u_{1}, u_{t}) + d (u_{2}, u_{t}) + \dots + d (u_{t}, u_{t})] \\ = & \frac{1}{2} [(t - 1) + (t - 1) + \dots + (t - 1)] \\ = & \frac{1}{2} [t (t - 1)] \end{array}$ $\begin{array}{rcl} M_{1} (Γ_{G}^{Υ}) & = & \sum_{i = 1}^{t} (d e g (u_{i}))^{2} \\ = & d e g (u_{1})^{2} + d e g (u_{2})^{2} + \dots + d e g (u_{t})^{2} \\ = & [(t - 1)^{2} + (t - 1)^{2} + \dots + (t - 1)^{2}] \\ = & t (t - 1)^{2} \end{array}$ $\begin{array}{rcl} M_{2} (Γ_{G}^{Υ}) & = & \sum_{i, j = 1}^{\frac{t (t - 1)}{2}} d e g (u_{i}) d e g (u_{j}) \\ = & (t - 1) (t - 1) + (t - 1) (t - 1) + \dots + (t - 1) (t - 1) \\ = & (t - 1) (t - 1) (\frac{t (t - 1)}{2}) \\ = & (t - 1)^{3} \frac{t}{2} \end{array}$ $\begin{array}{rcl} M_{3} (Γ_{G}^{Υ}) & = & \sum_{i, j = 1}^{\frac{t (t - 1)}{2}} (d e g (u_{i}) d e g (u_{j})) (d e g (u_{i}) + d e g (u_{j})) \\ = & [((t - 1) (t - 1)) ((t - 1) + (t - 1))] + \\ [((t - 1) (t - 1)) ((t - 1) + (t - 1))] + \dots + \\ [((t - 1) (t - 1)) ((t - 1) + (t - 1))] \\ = & (t - 1)^{2} [2 (t - 1)] + (t - 1)^{2} [2 (t - 1)] + \dots + \\ (t - 1)^{2} [2 (t - 1)] \\ = & 2 (t - 1)^{3} + 2 (t - 1)^{3} + \dots + 2 (t - 1)^{3} \\ = & \frac{t (t - 1)}{2} [2 (t - 1)^{3}] \\ = & t (t - 1)^{4} \end{array}$ $\begin{array}{rcl} H M_{1} (Γ_{G}^{Υ}) & = & \sum_{i, j = 1}^{\frac{t (t - 1)}{2}} (d e g (u_{i}) + d e g (u_{j}))^{2} \\ = & ((t - 1) + (t - 1))^{2} + ((t - 1) + (t - 1))^{2} + \dots + \\ ((t - 1) + (t - 1))^{2} \\ = & (2 (t - 1))^{2} + (2 (t - 1))^{2} + \dots + (2 (t - 1))^{2} \\ = & \frac{t (t - 1)}{2} (2 (t - 1))^{2} \\ = & \frac{t (t - 1)}{2} [4 (t - 1)^{2}] \\ = & 2 t (t - 1)^{3} \end{array}$ $\begin{array}{rcl} H M_{2} (Γ_{G}^{Υ}) & = & \sum_{i, j = 1}^{\frac{t (t - 1)}{2}} (d e g (u_{i}) d e g (u_{j}))^{2} \\ = & ((t - 1) (t - 1))^{2} + ((t - 1) (t - 1))^{2} + \dots + \\ ((t - 1) (t - 1))^{2} \\ = & ((t - 1)^{2})^{2} + ((t - 1)^{2})^{2} + \dots + ((t - 1)^{2})^{2} \\ = & (t - 1)^{4} + (t - 1)^{4} + \dots + (t - 1)^{4} \\ = & \frac{t (t - 1)}{2} (t - 1)^{4} \\ = & \frac{t (t - 1)^{5}}{2} \end{array}$ $\begin{array}{rcl} M o d M_{1} (Γ_{G}^{Υ}) & = & \sum_{i, j = 1}^{t} \frac{1}{d e g (u_{i})^{2}} \\ = & \frac{1}{(t - 1)^{2}} + \frac{1}{(t - 1)^{2}} + \dots + \frac{1}{(t - 1)^{2}} \\ = & t [\frac{1}{(t - 1)^{2}}] \\ = & \frac{t}{(t - 1)^{2}} \end{array}$ $\begin{array}{rcl} S_{c} (Γ_{G}^{Υ}) & = & \frac{1}{2} \sum (d e g (u_{i}) + d e g (u_{j})) d (u_{i}, u_{j}) \\ = & \frac{1}{2} [((t - 1) + (t - 1)) (t - 1) + ((t - 1) + (t - 1)) \\ (t - 1) + \dots + ((t - 1) + (t - 1)) (t - 1)] \\ = & \frac{1}{2} [2 (t - 1)^{2} + 2 (t - 1)^{2} + \dots + 2 (t - 1)^{2}] \\ = & \frac{1}{2} t (2 (t - 1)^{2}) \\ = & t (t - 1)^{2} \end{array}$ $\begin{array}{rcl} S_{c}^{*} (Γ_{G}^{Υ}) & = & \frac{1}{2} \sum (d e g (u_{i}) d e g (u_{j})) d (u_{i}, u_{j}) \\ = & \frac{1}{2} [((t - 1) (t - 1)) (t - 1) + ((t - 1) (t - 1)) (t - 1) \\ + \dots + ((t - 1) (t - 1)) (t - 1)] \\ = & \frac{1}{2} [(t - 1)^{3} + (t - 1)^{3} + \dots + (t - 1)^{3}] \\ = & \frac{1}{2} t (t - 1)^{3} \end{array}$ $\begin{array}{rcl} F (Γ_{G}^{Υ}) & = & \sum_{i, j = 1}^{\frac{t (t - 1)}{2}} d e g (u_{i})^{2} + d e g (u_{j})^{2} \\ = & ((t - 1)^{2} + (t - 1)^{2}) + ((t - 1)^{2} + (t - 1)^{2}) \\ + \dots + ((t - 1)^{2} + (t - 1)^{2}) \\ = & [2 (t - 1)^{2} + 2 (t - 1)^{2} + \dots + 2 (t - 1)^{2}] \\ = & \frac{t (t - 1)}{2} [2 (t - 1)^{2}] \\ = & t (t - 1)^{3} \end{array}$ $\begin{array}{rcl} S_{o} (Γ_{G}^{Υ}) & = & \sum_{i, j = 1}^{\frac{t (t - 1)}{2}} (d e g (u_{i})^{2} + d e g (u_{j})^{2})^{\frac{1}{2}} \\ = & ((t - 1)^{2} + (t - 1)^{2})^{\frac{1}{2}} + ((t - 1)^{2} + (t - 1)^{2})^{\frac{1}{2}} \\ + \dots + ((t - 1)^{2} + (t - 1)^{2})^{\frac{1}{2}} \\ = & (2 (t - 1)^{2})^{\frac{1}{2}} + (2 (t - 1)^{2})^{\frac{1}{2}} + \dots + (2 (t - 1)^{2})^{\frac{1}{2}} \\ = & 2 (t - 1) + 2 (t - 1) + \dots + 2 (t - 1) \\ = & \frac{t (t - 1)}{2} (2 (t - 1)) \\ = & t (t - 1)^{2} \end{array}$ $\begin{array}{rcl} R (Γ_{G}^{Υ}) & = & \sum_{i, j = 1}^{\frac{t (t - 1)}{2}} (d e g (u_{i}) d e g (u_{j})) \frac{- 1}{2} \\ = & \sum_{i, j = 1}^{\frac{t (t - 1)}{2}} \frac{1}{\sqrt{d e g (u_{i}) d e g (u_{j})}} \\ = & \frac{1}{((t - 1) (t - 1))^{\frac{1}{2}}} + \frac{1}{((t - 1) (t - 1))^{\frac{1}{2}}} + \dots + \\ \frac{1}{((t - 1) (t - 1))^{\frac{1}{2}}} \\ = & \frac{1}{((t - 1)^{2})^{\frac{1}{2}}} + \frac{1}{((t - 1)^{2})^{\frac{1}{2}}} + \dots + \frac{1}{((t - 1)^{2})^{\frac{1}{2}}} \\ = & \frac{1}{(t - 1)} + \frac{1}{(t - 1)} + \dots + \frac{1}{(t - 1)} \end{array}$ $\begin{array}{rcl} = & \frac{t (t - 1)}{2} (\frac{1}{(t - 1)}) \\ = & \frac{t}{2} \end{array}$ $\begin{array}{rcl} R R (Γ_{G}^{Υ}) & = & \sum_{i, j = 1}^{\frac{t (t - 1)}{2}} (d e g (u_{i}) d e g (u_{j}))^{\frac{1}{2}} \\ = & ((t - 1) (t - 1))^{\frac{1}{2}} + ((t - 1) (t - 1))^{\frac{1}{2}} + \dots + \\ ((t - 1) (t - 1))^{\frac{1}{2}} \\ = & ((t - 1)^{2})^{\frac{1}{2}} + ((t - 1)^{2})^{\frac{1}{2}} + \dots + ((t - 1)^{2})^{\frac{1}{2}} \\ = & \frac{t (t - 1)}{2} [((t - 1)^{2}) \frac{1}{2}] \\ = & \frac{t (t - 1)^{2}}{2} \end{array}$

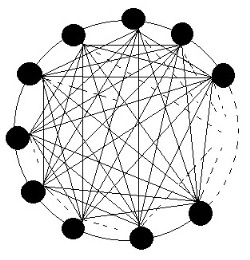
Example 1: Consider the orbit graph $K_{15}$ (see Figure $2$ ).
Now, the graph $K_{15}$ have $| Υ | - | B | = 15 - 0 = 15$ vertices and $\frac{15 (15 - 1)}{2} = 105$ edges, respectively.

Also, the degree and distance of vertices are $(15 - 1) = 14$ . Then $\begin{array}{rcl} W (Γ_{G}^{Υ}) & = & 105 \\ M_{1} (Γ_{G}^{Υ}) & = & 2940 \\ M_{2} (Γ_{G}^{Υ}) & = & 20580 \\ M_{3} (Γ_{G}^{Υ}) & = & 576240 \\ H M_{1} (Γ_{G}^{Υ}) & = & 82320 \\ H M_{2} (Γ_{G}^{Υ}) & = & 4033680 \\ M o d M_{1} (Γ_{G}^{Υ}) & = & 0.00510 \\ S_{c} (Γ_{G}^{Υ}) & = & 2940 \\ S_{c}^{*} (Γ_{G}^{Υ}) & = & 20580 \\ F (Γ_{G}^{Υ}) & = & 41160 \\ S_{o} (Γ_{G}^{Υ}) & = & 2940 \\ R (Γ_{G}^{Υ}) & = & 7.5 \\ R R (Γ_{G}^{Υ}) & = & 1470 \end{array}$

4. Relation with wiener index

In this section, we relate each of the indices with the Wiener index and also their relationship with each other.

Theorem 3. For orbit graph of dihedral group,
$S_{c}^{*} (Γ_{G}^{Υ}) = W (Γ_{G}^{Υ}) (t - 1)^{2} = M_{2} (Γ_{G}^{Υ})$

Proof. As we obtain the modified schultz index for the orbit graph of dihedral group in the above Theorem 2. Then we have
$S_{c}^{*} (Γ_{G}^{Υ}) = \frac{t (t - 1)^{3}}{2}$
Since, $W (Γ_{G}^{Υ})$ = $\frac{1}{2} t (t - 1)$ then by substituting the value of $W (Γ_{G}^{Υ})$ in $S_{c}^{*} (Γ_{G}^{Υ})$ ,we get $\begin{array}{rcl} S_{c}^{*} (Γ_{G}^{Υ}) = & \frac{t (t - 1)}{2} (t - 1)^{2} \\ = & W (Γ_{G}^{Υ}) (t - 1)^{2} \end{array}$ This implies,
$S_{c}^{*} (Γ_{G}^{Υ})$ = $W (Γ_{G}^{Υ}) (t - 1)^{2}$ .
and it is obvious from the above prove result that $S_{c}^{*} (Γ_{G}^{Υ}) = M_{2} (Γ_{G}^{Υ})$ .

Theorem 4. $M_{1} (Γ_{G}^{Υ}) = S_{c} (Γ_{G}^{Υ}) = S_{o} (Γ_{G}^{Υ}) = 2 (t - 1) W (Γ_{G}^{Υ})$

Proof. Since, $\begin{array}{rcl} M_{1} (Γ_{G}^{Υ}) = & t (t - 1)^{2} \\ S_{c} (Γ_{G}^{Υ}) = & t (t - 1)^{2} \\ S_{o} (Γ_{G}^{Υ}) = & t (t - 1)^{2} \end{array}$ This implies, $\begin{array}{rcl} W (Γ_{G}^{Υ}) = & \frac{1}{2} t (t - 1) \\ 2 W (Γ_{G}^{Υ}) = & t (t - 1) \end{array}$ Therefore,
$M_{1} (Γ_{G}^{Υ}) = S_{c} (Γ_{G}^{Υ}) = S_{o} (Γ_{G}^{Υ}) = 2 (t - 1) W (Γ_{G}^{Υ})$

Theorem 5. For orbit graph of dihedral group, the second Zagreb index is the product of Wiener index and the first Zagreb index. $M_{2} (Γ_{G}^{Υ}) = \frac{1}{t} W (Γ_{G}^{Υ}) M_{1} (Γ_{G}^{Υ})$

Proof. we obtain $M_{2} (Γ_{G}^{Υ})$ of dihedral group as
$M_{2} (Γ_{G}^{Υ}) = \frac{t (t - 1)^{3}}{2}$ Then, $\begin{array}{rcl} M_{2} (Γ_{G}^{Υ}) = & t (t - 1)^{2} \frac{(t - 1)}{2} \\ = & \frac{1}{2} (t - 1) M_{1} (Γ_{G}^{Υ}) \\ = & \frac{1}{t} W (Γ_{G}^{Υ}) M_{1} (Γ_{G}^{Υ}) \end{array}$

Theorem 6. $M_{3} (Γ_{G}^{Υ}) = (t - 1)^{2} M_{1} (Γ_{G}^{Υ}) = \frac{4}{t} W (Γ_{G}^{Υ}) M_{2} (Γ_{G}^{Υ})$

Proof. $\begin{array}{rcl} M_{3} (Γ_{G}^{Υ}) = & t (t - 1)^{4} \\ = & t (t - 1)^{2} (t - 1)^{2} \\ = & (t - 1)^{2} M_{1} (Γ_{G}^{Υ}) \end{array}$ Again, $\begin{array}{rcl} M_{3} (Γ_{G}^{Υ}) = & t (t - 1)^{4} \\ = & t (t - 1)^{3} (t - 1) \\ = & 2 (t - 1) M_{2} (Γ_{G}^{Υ}) \\ = & \frac{4}{t} W (Γ_{G}^{Υ}) M_{2} (Γ_{G}^{Υ}) \end{array}$ where, $(t - 1) = \frac{2}{t} W (Γ_{G}^{Υ})$ and $t (t - 1)^{3} = 2 M_{2} (Γ_{G}^{Υ})$ .

Theorem 7. $H M_{1} (Γ_{G}^{Υ}) = 2 (t - 1) M_{1} (Γ_{G}^{Υ}) = \frac{4}{t} W (Γ_{G}^{Υ}) M_{1} (Γ_{G}^{Υ}) = 4 (t - 1)^{2} W (Γ_{G}^{Υ})$ .

Proof. $\begin{array}{rcl} H M_{1} (Γ_{G}^{Υ}) = & 2 t (t - 1)^{3} \\ = & 2 (t - 1) t (t - 1)^{2} \\ = & 2 (t - 1) M_{1} (Γ_{G}^{Υ}) \\ = & \frac{4}{t} W (Γ_{G}^{Υ}) M_{1} (Γ_{G}^{Υ}) \end{array}$ where, $(t - 1) = \frac{2}{t} W (Γ_{G}^{Υ})$ .
Also, $\begin{array}{rcl} H M_{1} (Γ_{G}^{Υ}) = & 2 t (t - 1)^{3} \\ = & 2 t (t - 1) (t - 1)^{2} \\ = & 4 (t - 1)^{2} W (Γ_{G}^{Υ}) \end{array}$ where, $t (t - 1) = 2 W (Γ_{G}^{Υ})$ .

Theorem 8. $H M_{2} (Γ_{G}^{Υ}) = (t - 1)^{4} W (Γ_{G}^{Υ}) = \frac{1}{2} (t - 1)^{3} M_{1} (Γ_{G}^{Υ}) = (t - 1)^{2} M_{2} (Γ_{G}^{Υ})$ .

Proof. By splitting the $H M_{2} (Γ_{G}^{Υ}) = \frac{t (t - 1)^{5}}{2}$ , we obtain the result

Theorem 9. $M o d M_{1} (Γ_{G}^{Υ}) = \frac{2}{(t - 1)^{2}} R (Γ_{G}^{Υ})$

Proof. From Theorem 2, we obtain $\begin{array}{rcl} M o d M_{1} (Γ_{G}^{Υ}) = & \frac{t}{(t - 1)^{2}} \end{array}$ and $\begin{array}{rcl} R (Γ_{G}^{Υ}) = & \frac{t}{2} \end{array}$ This implies, $\begin{array}{rcl} 2 R (Γ_{G}^{Υ}) = & t \end{array}$ Substituting the value of $t$ in $M o d M_{1} (Γ_{G}^{Υ})$ , we have $\begin{array}{rcl} M o d M_{1} (Γ_{G}^{Υ}) = & \frac{2 R (Γ_{G}^{Υ})}{(t - 1)^{2}} \end{array}$

Theorem 10. For the orbit graph of dihedral group, Forgotten index is the twice of second Zagreb index and modified Schultz index $\begin{array}{rcl} F (Γ_{G}^{Υ}) & = & 2 M_{2} (Γ_{G}^{Υ}) = 2 S_{c}^{*} (Γ_{G}^{Υ}) \\ F (Γ_{G}^{Υ}) & = & 2 (t - 1)^{2} W (Γ_{G}^{Υ}) = (t - 1) M_{1} (Γ_{G}^{Υ}) = 2 (t - 1) R R (Γ_{G}^{Υ}) . \end{array}$

Proof. From Theorem 2, $F (Γ_{G}^{Υ}) = 2 M_{2} (Γ_{G}^{Υ}) = 2 S_{c}^{*} (Γ_{G}^{Υ})$ .
Now,
$F (Γ_{G}^{Υ}) = t (t - 1)^{3}$ by splitting the formula as $t (t - 1)^{2} (t - 1)$ , we have
$F (Γ_{G}^{Υ}) = (t - 1) M_{1} (Γ_{G}^{Υ})$
Since, $W (Γ_{G}^{Υ}) = \frac{1}{2} t (t - 1)$ which gives $F (Γ_{G}^{Υ}) = 2 (t - 1)^{2} W (Γ_{G}^{Υ})$
Also, $\begin{array}{rcl} F (Γ_{G}^{Υ}) = & t (t - 1)^{3} \\ = & t (t - 1)^{2} (t - 1) \\ = & 2 (t - 1) R R (Γ_{G}^{Υ}) \end{array}$ where, $t (t - 1)^{2} = 2 R R (Γ_{G}^{Υ})$ .

5. Polynomial for the Indices of $D_{t}$

In this section, we obtain the polynomial of each index for the orbit graph of the dihedral group.

Theorem 11. Let $Γ_{G}^{Υ}$ be the orbit graph of the dihedral group. Then the polynomial of each index is

$W (Γ_{G}^{Υ}) = \sum x^{\frac{1}{2} t (t - 1)}$
$M_{1} (Γ_{G}^{Υ}) = \sum x^{t (t - 1)^{2}}$
$M_{2} (Γ_{G}^{Υ}) = \sum x^{\frac{t (t - 1)^{3}}{2}}$
$M_{3} (Γ_{G}^{Υ}) = \sum x^{t (t - 1)^{4}}$
$H M_{1} (Γ_{G}^{Υ}) = \sum x^{2 t (t - 1)^{3}}$
$H M_{2} (Γ_{G}^{Υ}) = \sum x^{\frac{t (t - 1)^{5}}{2}}$
$M o d M_{1} (Γ_{G}^{Υ}) = \sum x^{\frac{t}{(t - 1)^{2}}}$
$S_{c} (Γ_{G}^{Υ}) = \sum x^{t (t - 1)^{2}}$
$S_{c}^{*} (Γ_{G}^{Υ}) = \sum x^{\frac{t (t - 1)^{3}}{2}}$
$F (Γ_{G}^{Υ}) = \sum x^{t (t - 1)^{3}}$
$S_{o} (Γ_{G}^{Υ}) = \sum x^{t (t - 1)^{2}}$
$R (Γ_{G}^{Υ}) = \sum x^{\frac{t}{2}}$
$R R (Γ_{G}^{Υ}) = \sum x^{\frac{t (t - 1)^{2}}{2}}$

Proof. From Theorem 2, the result is obvious.

Example 2: Consider the orbit graph $K_{9}$ (see Figure $3$ ).
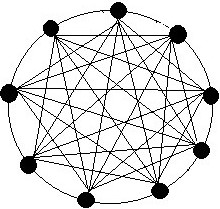
Now, the graph $K_{9}$ have $| Υ | - | B | = 9 - 0 = 9$ vertices and $\frac{9 (9 - 1)}{2} = 36$ edges, respectively.

Also, the degree and distance of vertices are $(9 - 1) = 8$ . Then $\begin{array}{rcl} W (Γ_{G}^{Υ}) = & \sum x^{36} \\ M_{1} (Γ_{G}^{Υ}) = & \sum x^{576} \\ M_{2} (Γ_{G}^{Υ}) = & \sum x^{2304} \\ M_{3} (Γ_{G}^{Υ}) = & \sum x^{36864} \\ H M_{1} (Γ_{G}^{Υ}) = & \sum x^{9216} \\ H M_{2} (Γ_{G}^{Υ}) = & \sum x^{147456} \\ M o d M_{1} (Γ_{G}^{Υ}) = & \sum x^{0.14} \\ S_{c} (Γ_{G}^{Υ}) = & \sum x^{576} \\ S_{c}^{*} (Γ_{G}^{Υ}) = & \sum x^{2304} \\ F (Γ_{G}^{Υ}) = & \sum x^{4608} \\ S_{o} (Γ_{G}^{Υ}) = & \sum x^{576} \\ R (Γ_{G}^{Υ}) = & \sum x^{4.5} \\ R R (Γ_{G}^{Υ}) = & \sum x^{288} \end{array}$

6. Conclusion

Because topological indices can only be computed for connected graphs, we only consider the third case of the dihedral group’s orbit graph. Since the orbit graph of a dihedral group is complete, we obtain the indices by generalising the Wiener index, the first, second and third Zagreb indices, the Hyper first and second Zagreb indices, the Schultz and modified Schultz indices, the Somber index, the Forgotten index, and the Randic and Reciprocal Randic indices of a complete graph. We also learn how these indices relate to the Wiener index for the orbit graph of the dihedral group. Furthermore, the polynomial for the indices of the group’s orbit graph has been determined.

Acknowledgement

The authors would like to express their sincere thanks to referee(s) for comments and remarks.

References:

Stankevich, M.I., Stankevich, I.V. and Zefirov, N.S., 1988. Topological indices in organic chemistry. Russian Chemical Reviews, 57(3), p.191.[Google Scholor]
Diudea, M.V. and Gutman, I., 1998. Wiener-type topological indices. Croatica chemica acta, 71(1), pp.21-51.[Google Scholor]
Cancan, M., Ediz, S., Fareed, S. and Farahani, M.R., 2020. More topological indices of generalized prism network. Journal of Information and Optimization Sciences, 41(4), pp.925-932.[Google Scholor]
Gutman, I. and Trinajstic, N., 1972. Graph theory and molecular orbitals. Total $π$ -electron energy of alternant hydrocarbons. Chemical physics letters, 17(4), pp.535-538.[Google Scholor]
Das, K.C. and Gutman, I., 2004. Some properties of the second Zagreb index. MATCH Communications in Mathematical and in Computer Chemistry, 52(1), p.31.[Google Scholor]
Munir, M., Nazeer, W., Rafique, S. and Kang, S.M., 2016. M-polynomial and related topological indices of nanostar dendrimers. Symmetry, 8(9), p.97.[Google Scholor]
Zhou, B. and Gutman, I., 2004. Relations between Wiener, hyper-Wiener and Zagreb indices. Chemical Physics Letters, 394(1-3), pp.93-95.[Google Scholor]
Khalifeh, M.H., Yousefi-Azari, H. and Ashrafi, A.R., 2009. The first and second Zagreb indices of some graph operations. Discrete applied mathematics, 157(4), pp.804-811.[Google Scholor]
Khalaf, A.J.M., Hanif, M.F., Siddiqui, M.K. and Farahani, M.R., 2020. On degree based topological indices of bridge graphs. Journal of Discrete Mathematical Sciences and Cryptography, 23(6), pp.1139-1156.[Google Scholor]
Das, K.C., Jeon, H.U. and Trinajsti{\’c}, N., 2014. Comparison between the Wiener index and the Zagreb indices and the eccentric connectivity index for trees. Discrete Applied Mathematics, 171, pp.35-41.[Google Scholor]
Bello, M., Ali, N.M., Isah, S.I. and Zulkifli, N., 2020. The Wiener and Zagreb indices of the order product prime graph. Journal Critical Reviews., 7(16), pp.896-901.[Google Scholor]
Singh, P. and Bhat, V.K., 2021. Adjacency matrix and Wiener index of zero divisor graph $Γ (Z_{n})$ . Journal of Applied Mathematics and Computing, 66, pp.717-732.[Google Scholor]
Shahistha, K., Bhat, A. and Sudhakara, G., Wiener index of chain graphs. IAENG International Journal of Applied Mathematics, 50(4).[Google Scholor]
Poojary, P., Raghavendra, A., Shenoy, B.G., Farahani, M.R. and Sooryanarayana, B., 2021. Certain topological indices and polynomials for the Isaac graphs. Journal of Discrete Mathematical Sciences and Cryptography, 24(2), pp.511-525.[Google Scholor]
Filipovski, S., 2021. Relations between Sombor index and some degree-based topological indices. Iranian Journal of Mathematical Chemistry, 12(1), pp.19-26.[Google Scholor]
Al-Ahmadi, B., Saleh, A. and Al-Shammakh, W., 2021. Downhill Zagreb Topological Indices of Graphs. International Journal of Analysis and Applications, 19(2), pp.205-227.[Google Scholor]
Saeed, N. Long, K. Mufti, Z. S. Sajid, H. and Rehman, A., 2021. Degree Based topological indices of Boron $B_{12}$ . Journal of Chemistry, p.5563218.[Google Scholor]
Javaid, M., Ali, U. and Siddiqui, K., 2021. Novel connection based Zagreb indices of several wheel-related graphs. Computational Journal of Combinatorial Mathematics, 1, pp.1-28.[Google Scholor]
Saleh, A., Alqesmah, A., Alashwali, H. and Cangul, I.N., 2022. Entire Wiener index of graphs. Communications in Combinatorics & Optimization, 7(2), 1 – 19.[Google Scholor]
Sarmin, N.H., El-sanfaz, M.A. and Omer, S.M.S., 2016, June. Groups and graphs in probability theory. In AIP Conference Proceedings (Vol. 1750, No. 1). AIP Publishing.[Google Scholor]
Bondy, J. and Murty, U., 1976. Graph Theory with Applications, The Macmillan Press LTD. (1976).[Google Scholor]
Alimon, N.I., Sarmin, N.H. and Erfanian, A., 2019. The Wiener and Zagreb indices of conjugacy class graph of the dihedral groups. MATEMATIKA: Malaysian Journal of Industrial and Applied Mathematics, pp.51-57.[Google Scholor]

[1] Stankevich, M.I., Stankevich, I.V. and Zefirov, N.S., 1988. Topological indices in organic chemistry. Russian Chemical Reviews, 57(3), p.191.[Google Scholor]

[2] Diudea, M.V. and Gutman, I., 1998. Wiener-type topological indices. Croatica chemica acta, 71(1), pp.21-51.[Google Scholor]

[3] Cancan, M., Ediz, S., Fareed, S. and Farahani, M.R., 2020. More topological indices of generalized prism network. Journal of Information and Optimization Sciences, 41(4), pp.925-932.[Google Scholor]

[4] Gutman, I. and Trinajstic, N., 1972. Graph theory and molecular orbitals. Total $π$ -electron energy of alternant hydrocarbons. Chemical physics letters, 17(4), pp.535-538.[Google Scholor]

[5] Das, K.C. and Gutman, I., 2004. Some properties of the second Zagreb index. MATCH Communications in Mathematical and in Computer Chemistry, 52(1), p.31.[Google Scholor]

[6] Munir, M., Nazeer, W., Rafique, S. and Kang, S.M., 2016. M-polynomial and related topological indices of nanostar dendrimers. Symmetry, 8(9), p.97.[Google Scholor]

[7] Zhou, B. and Gutman, I., 2004. Relations between Wiener, hyper-Wiener and Zagreb indices. Chemical Physics Letters, 394(1-3), pp.93-95.[Google Scholor]

[8] Khalifeh, M.H., Yousefi-Azari, H. and Ashrafi, A.R., 2009. The first and second Zagreb indices of some graph operations. Discrete applied mathematics, 157(4), pp.804-811.[Google Scholor]

[9] Khalaf, A.J.M., Hanif, M.F., Siddiqui, M.K. and Farahani, M.R., 2020. On degree based topological indices of bridge graphs. Journal of Discrete Mathematical Sciences and Cryptography, 23(6), pp.1139-1156.[Google Scholor]

[10] Das, K.C., Jeon, H.U. and Trinajsti{\’c}, N., 2014. Comparison between the Wiener index and the Zagreb indices and the eccentric connectivity index for trees. Discrete Applied Mathematics, 171, pp.35-41.[Google Scholor]

[11] Bello, M., Ali, N.M., Isah, S.I. and Zulkifli, N., 2020. The Wiener and Zagreb indices of the order product prime graph. Journal Critical Reviews., 7(16), pp.896-901.[Google Scholor]

[12] Singh, P. and Bhat, V.K., 2021. Adjacency matrix and Wiener index of zero divisor graph $Γ (Z_{n})$ . Journal of Applied Mathematics and Computing, 66, pp.717-732.[Google Scholor]

[13] Shahistha, K., Bhat, A. and Sudhakara, G., Wiener index of chain graphs. IAENG International Journal of Applied Mathematics, 50(4).[Google Scholor]

[14] Poojary, P., Raghavendra, A., Shenoy, B.G., Farahani, M.R. and Sooryanarayana, B., 2021. Certain topological indices and polynomials for the Isaac graphs. Journal of Discrete Mathematical Sciences and Cryptography, 24(2), pp.511-525.[Google Scholor]

[15] Filipovski, S., 2021. Relations between Sombor index and some degree-based topological indices. Iranian Journal of Mathematical Chemistry, 12(1), pp.19-26.[Google Scholor]

[16] Al-Ahmadi, B., Saleh, A. and Al-Shammakh, W., 2021. Downhill Zagreb Topological Indices of Graphs. International Journal of Analysis and Applications, 19(2), pp.205-227.[Google Scholor]

[17] Saeed, N. Long, K. Mufti, Z. S. Sajid, H. and Rehman, A., 2021. Degree Based topological indices of Boron $B_{12}$ . Journal of Chemistry, p.5563218.[Google Scholor]

[18] Javaid, M., Ali, U. and Siddiqui, K., 2021. Novel connection based Zagreb indices of several wheel-related graphs. Computational Journal of Combinatorial Mathematics, 1, pp.1-28.[Google Scholor]

[19] Saleh, A., Alqesmah, A., Alashwali, H. and Cangul, I.N., 2022. Entire Wiener index of graphs. Communications in Combinatorics & Optimization, 7(2), 1 – 19.[Google Scholor]

[20] Sarmin, N.H., El-sanfaz, M.A. and Omer, S.M.S., 2016, June. Groups and graphs in probability theory. In AIP Conference Proceedings (Vol. 1750, No. 1). AIP Publishing.[Google Scholor]

[21] Bondy, J. and Murty, U., 1976. Graph Theory with Applications, The Macmillan Press LTD. (1976).[Google Scholor]

[22] Alimon, N.I., Sarmin, N.H. and Erfanian, A., 2019. The Wiener and Zagreb indices of conjugacy class graph of the dihedral groups. MATEMATIKA: Malaysian Journal of Industrial and Applied Mathematics, pp.51-57.[Google Scholor]

Contents

Journal of Combinatorial Mathematics and Combinatorial Computing