Let \(G\) be a \((p, q)\) graph. Let \(f: V(G) \to \{1, 2, \ldots, k\}\) be a map where \(k \in \mathbb{N}\) is a variable and \(k > 1\). For each edge \(uv\), assign the label \(\gcd(f(u), f(v))\). \(f\) is called \(k\)-Total prime cordial labeling of \(G\) if \(\left|t_{f}(i) – t_{f}(j)\right| \leq 1\), \(i, j \in \{1, 2, \ldots, k\}\) where \(t_{f}(x)\) denotes the total number of vertices and edges labeled with \(x\). A graph with a \(k\)-total prime cordial labeling is called \(k\)-total prime cordial graph. In this paper, we investigate the 4-total prime cordial labeling of some graphs like dragon, Möbius ladder, and corona of some graphs.
Graphs considered here are finite, simple and undirected. A weaker version of graceful and harmonious labeling called cordial labeling was introduced by cahit [1]. Subsequently cordial related labeling have been studied by several author [2-4]. Ponraj et al. [5], have been introduced the notion of \(k\)-total prime cordial labeling and the \(k\)-total prime cordial labeling of certain graphs have been investigated. In [5-9], the \(4\)-total prime cordial labeling behaviour of path, cycle, star, bistar, some complete graphs, comb, double comb, triangular snake, double triangular snake, ladder, friendship graph, flower graph, gear graph, Jelly fish, book, irregular triangular snake, prism, helm, dumbbell graph, sunflower graph, corona of irregular triangular snake, corona of some graphs and subdivision of some graphs. \(3\)-total prime cordial labeling behaviour of some graphs have been investigated []. In this paper we investigate the \(4\)-total prime cordial labeling of few graphs like dragon, mobius ladder and corona of some graphs.
Definition 1. Let \(G_{1}\), \(G_{2}\) respectively be \((p_{1}, q_{1})\), \((p_{2}, q_{2})\) graphs. The corona of \(G_{1}\) with \(G_{2}\) is the graph \(G_{1} \odot G_{2}\) obtained by taking one copy of \(G_{1}\) , \(p_{1}\) copies of \(G_{2}\) and joining the \(i^{th}\) vertex of \(G_{1}\) by an edge to every vertex in the \(i^{th}\) copy of \(G_{2}\) where \(1 \leq i \leq p_1\).
Definition 2. The cartesian product of two graphs \(G_1\) and \(G_2\) is the graph \(G_{1}\times G_{2}\) with vertex set \(V_{1}\times V_{2}\) and two vertices \(u=(u_1,u_2)\) and \(v=(v_1,v_2)\) are adjacent whenever \([u_1=v_1\) and \(u_2\) adj \(v_2]\) or \([u_2=v_2\) and \(u_1\) adj \(v_1]\).
Definition 3. An n-sided prism \(Pr_n\) is a planar graph having 2 faces viz., an inner face and outer face with n sides and every other face is a 4-cycle. In other words, it is \(C_n \times K_2.\)
Definition 4. The mobius ladder \(M_{n}\) is the graph obtained from the ladder \(P_{n} \times P_{2}\) by joining the opposite end points of the two copies of \(P_{n}\).
Theorem 1. [5] All paths are \(4\)-total prime cordial.
For the purpose of the main section we once again recall the \(4\)-total prime cordial labeling of the path:
For \(n=4r\), \(r>1\) and \(r \in \mathbb{N}\). The labeling pattern is given in Table 1.
| Vertices | \(u_{1},\ldots,u_{r}\) | \(u_{r+1},\ldots,u_{2r}\) | \(u_{2r+1},\ldots,u_{3r}\) | \(u_{3r+1},\ldots,u_{4r-1}\) | \(u_{4r}\) |
|---|---|---|---|---|---|
| Labels | \(4\) | \(2\) | \(3\) | \(1\) | \(3\) |
For \(n=4r+1\), \(r>1\) and \(r
\in \mathbb{N}\). Label the vertices \(u_{i}\) \((1 \leq
i \leq 4r)\) as above pattern in Table 1. Assign the label
\(4\) to the vertex \(u_{4r+1}\).
For \(n=4r+2\), \(r>1\) and \(r
\in \mathbb{N}\). The labeling pattern is given in Table 2 and
Table 3
| Vertices | \(u_{1},\ldots,u_{r+1}\) | \(u_{r+2},\ldots,u_{2r+1}\) | \(u_{2r+2},\ldots,u_{3r+1}\) |
|---|---|---|---|
| Labels | \(4\) | \(2\) | \(3\) |
| Vertices | \(u_{3r+2},\ldots,u_{4r}\) | \(u_{4r+1}\) | \(u_{4r+2}\) |
|---|---|---|---|
| Labels | \(1\) | \(3\) | \(2\) |
For \(n=4r+3\), \(r>1\) and \(r \in \mathbb{N}\). Label the vertices \(u_{i}\) \((1 \leq i \leq 4r+2)\) as above pattern in Table 1. Assign the label \(3\) to the vertex \(u_{4r+3}\).
A \(4\)-total prime cordial labeling of \(P_{n}\), \(n=3\), \(4\), \(5\), \(6\), \(7\) is given in Table 4.
| n | \(u_{1}\) | \(u_{2}\) | \(u_{3}\) | \(u_{4}\) | \(u_{5}\) | \(u_{6}\) | \(u_{7}\) |
|---|---|---|---|---|---|---|---|
| 3 | 4 | 2 | 3 | ||||
| 4 | 4 | 2 | 3 | 4 | |||
| 5 | 4 | 3 | 2 | 4 | 3 | ||
| 6 | 4 | 3 | 4 | 2 | 4 | 3 | |
| 7 | 4 | 1 | 4 | 2 | 4 | 3 | 3 |
Theorem 2. ] [5] The cycle \(C_{n}\) is 4-total prime cordial iff \(n\notin\{4,6,8\}\).
When \(n=4r\), \(r>1\) and \(r \in \mathbb{N}\). Assign the same labeling pattern of \(P_{4r}\) \(u_{i}\) \(( 1 \leq i \leq n)\) given in Theorem 1. Next we relabel the vertex \(u_{4r-1}\) by \(4\).
When \(n=4r+1\), \(r>1\) and \(r \in \mathbb{N}\). The same labeling pattern of \(P_{4r+1}\) given in Theorem 1 is also a \(4\)-total prime cordial labeling of cycle \(C_{4r+1}\).
When \(n=4r+2\), \(r>1\) and \(r \in \mathbb{N}\). Assign the same labeling pattern of \(P_{4r+2}\) \(u_{i}\) \(( 1 \leq i \leq n)\) given in Theorem 1. Next we relabel the vertices \(u_{3r+2}\), \(u_{4r+1}\), \(u_{4r+2}\) respectively by \(3\), \(2\) and \(1\).
When \(n=4r+3\), \(r>1\) and \(r \in \mathbb{N}\). The same labeling pattern of \(P_{4r+3}\) \((1 \leq i \leq 4r)\) is given in Theorem 1. Finally we assign the labels to the vertices \(u_{4r+1}\), \(u_{4r+2}\), \(u_{4r+3}\) respectively by \(1\), \(3\) and \(2\).
A \(4\)-total prime cordial labeling of \(C_{n}\), \(n=3\), \(5\), \(7\) is given in Table 5.
| \(n\) | \(u_{1}\) | \(u_{2}\) | \(u_{3}\) | \(u_{4}\) | \(u_{5}\) | \(u_{6}\) | \(u_{7}\) |
|---|---|---|---|---|---|---|---|
| 3 | 4 | 2 | 3 | ||||
| 5 | 4 | 2 | 4 | 3 | 3 | ||
| 7 | 4 | 4 | 2 | 2 | 3 | 3 | 1 |
Remark 1. 2- total prime cordial graph is 2-total product cordial graph.
Definition 5. Let \(G\) be a \((p,q)\) graph. Let \(f:V(G)\to\{1,2, \ldots, k\}\) be a function where \(k \in \mathbb{N}\) is a variable and \(k>1\). For each edge \(uv\), assign the label \(\gcd(f(u)\), \(f(v))\). \(f\) is called \(k\)-Total prime cordial labeling of \(G\) if \(\left|t_{f}(i)-t_{f}(j)\right|\leq 1\), \(i,j \in \{1,2, \cdots,k\}\) where \(t_{f}(x)\) denotes the total number of vertices and the edges labelled with \(x\). A graph with a \(k\)-total prime cordial labeling is called \(k\)-total prime cordial graph.
First we investigate the \(4\)-total prime cordial labeling behavior of the mobius ladder.
Theorem 3. The mobius ladder \(M_{n}\) is \(4\)-total prime cordial iff \(n \neq 2, 3\).
Proof. Let \(V(L_{n})=\{u_{i}, v_{i} : 1 \leq i \leq n \}\) and \(E(L_{n})=\{u_{i}u_{i+1}, v_{i}v_{i+1} : 1 \leq i \leq n-1 \} \cup \{u_{i}v_{i} : 1 \leq i \leq n \} \cup \{u_{1}v_{n}, v_{1}u_{n} \}\). It is easy to verify that \(\left|V(M_{n})\right|+\left|E(M_{n})\right|=5n\).
Case 1. \(n\equiv 0\pmod4\).
Let \(n=4r\), \(r>1\) and \(r \in \mathbb{N}\). Assign the label \(4\) to the vertices \(u_{1},u_{2},\ldots,u_{r+1}\) and assign the label \(2\) to the vertices \(u_{r+2},u_{r+3},\ldots,u_{2r+1}\). Next we assign the label \(3\) to the vertices \(u_{2r+2},u_{2r+3},\ldots,u_{3r+1}\). Finally we assign the label \(1\) to the vertices \(u_{3r+2},u_{3r+3},\ldots,u_{4r}\). Next we consider the vertices \(v_{i}\) \((1 \leq i \leq n)\). Assign the label \(4\) to the vertices \(v_{1},v_{2},\ldots,v_{r}\) and assign the label \(2\) to the vertices \(v_{r+1},v_{r+2},\ldots,v_{2r}\) and next we assign the label \(3\) to the vertices \(v_{2r+1},v_{2r+2},\ldots,v_{3r+1}\). Finally we assign the label \(1\) to the vertices \(v_{3r+2},v_{3r+3},\ldots,v_{4r}\). Clearly \(t_{f}(1)=t_{f}(2)=t_{f}(3)=t_{f}(4)=5r\).
Case 2. \(n\equiv 1\pmod4\).
Let \(n=4r+1\), \(r>1\) and \(r \in \mathbb{N}\). As in case 1, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq n-1)\), \(v_{i}\) \((1 \leq i \leq n-2)\). Finally we assign the labels \(4\), \(2\), \(3\) respectively to the vertices \(u_{4r+1}\), \(v_{4r}\) and \(v_{4r+1}\). Here \(t_{f}(1)=t_{f}(2)=t_{f}(3)=5r+1\) and \(t_{f}(4)=5r+2\).
Case 3. \(n\equiv 2\pmod4\).
Let \(n=4r+2\), \(r>1\) and \(r \in \mathbb{N}\). As in case 1, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq n-2)\), \(v_{i}\) \((1 \leq i \leq n-2)\). Finally we assign the labels \(3\), \(4\), \(3\), \(2\) to the vertices \(u_{4r+1}\), \(u_{4r+2}\), \(v_{4r+1}\) and \(v_{4r+2}\) respectively. It is easy to verify that \(t_{f}(1)=t_{f}(4)=5r+2\) and \(t_{f}(2)=t_{f}(3)=5r+3\).
Case 4. \(n\equiv 3\pmod4\).
Let \(n=4r+3\), \(r>1\) and \(r \in \mathbb{N}\). Assign the label to the vertices \(u_{i}\) \((1 \leq i \leq n-4)\), \(v_{i}\) \((1 \leq i \leq n-4)\) by case 1. Finally we assign the labels \(3\), \(4\), \(3\), \(3\), \(2\), \(4\), \(2\), \(1\) respectively to the vertices \(u_{4r}\), \(u_{4r+1}\), \(u_{4r+2}\), \(u_{4r+3}\), \(v_{4r}\), \(v_{4r+1}\), \(v_{4r+2}\) and \(v_{4r+3}\) . Obviously \(t_{f}(1)=t_{f}(2)=t_{f}(3)=5r+4\) and \(t_{f}(4)=5r+3\).
Case 5. \(n=2\).
Suppose f is a \(4\)-total prime cordial labeling of \(M_{2}\) then any one of the following labeling pattern occurs:
\(t_{f}(1)=t_{f}(2)=3\) and \(t_{f}(3)=t_{f}(4)=2\).
\(t_{f}(1)=t_{f}(3)=3\) and \(t_{f}(2)=t_{f}(4)=2\).
\(t_{f}(1)=t_{f}(4)=3\) and \(t_{f}(2)=t_{f}(3)=2\).
\(t_{f}(2)=t_{f}(3)=3\) and \(t_{f}(1)=t_{f}(4)=2\).
\(t_{f}(2)=t_{f}(4)=3\) and \(t_{f}(1)=t_{f}(3)=2\).
\(t_{f}(3)=t_{f}(4)=3\) and \(t_{f}(1)=t_{f}(2)=2\).
To get the label \(4\), it should be a label of exactly two vertices this gives \(t_{f}(4)=3\). To get the label \(3\), it should be a label of exactly two vertices this gives \(t_{f}(3)=3\). Note that the case \(t_{f}(4)=2\) does not exists then \(t_{f}(2)=0\), a contradiction.
Case 6. \(n=3\).
Suppose f is a \(4\)-total prime cordial labeling of \(M_{3}\) then any one of the following labeling pattern occurs:
\(t_{f}(1)=t_{f}(2)=t_{f}(3)=4\) and \(t_{f}(4)=3\).
\(t_{f}(1)=t_{f}(2)=t_{f}(4)=4\) and \(t_{f}(3)=3\).
\(t_{f}(1)=t_{f}(3)=t_{f}(4)=4\) and \(t_{f}(2)=3\).
\(t_{f}(2)=t_{f}(3)=t_{f}(4)=4\) and \(t_{f}(1)=3\).
Clearly \(t_{f}(4) \neq 4\) and \(t_{f}(3) \neq 4\), a contradiction. Hence \(M_{3}\) does not admits a \(4\)-total prime cordial labeling.
Case 7. \(n=4,5,6,7\).
A \(4\)-total prime cordial labeling follows from Table 6.
| n | 4 | 5 | 6 | 7 |
|---|---|---|---|---|
| \(u_{1}\) | 4 | 4 | 4 | 4 |
| \(u_{2}\) | 4 | 4 | 4 | 4 |
| \(u_{3}\) | 2 | 2 | 2 | 2 |
| \(u_{4}\) | 3 | 4 | 3 | 3 |
| \(u_{5}\) | 2 | 3 | 3 | |
| \(u_{6}\) | 2 | 4 | ||
| \(u_{7}\) | 2 | |||
| \(v_{1}\) | 3 | 4 | 4 | 4 |
| \(v_{2}\) | 3 | 3 | 4 | 4 |
| \(v_{3}\) | 2 | 3 | 2 | 2 |
| \(v_{4}\) | 4 | 3 | 3 | 3 |
| \(v_{5}\) | 3 | 3 | 3 | |
| \(v_{6}\) | 1 | 1 | ||
| \(v_{7}\) | 3 |
◻
Next we investigate the \(4\)-total prime cordial labeling behavior of the dragon \(C_{m} @ P_{n}\).
Theorem 4. The dragon \(C_{m} @ P_{n}\) is \(4\)-total prime cordial if \(m,n \geq 8\).
Proof. Let \(C_{m}\) be the cycle \(u_{1}u_{2} \ldots u_{m}u_{1}\) and \(P_{n}\) be the path \(v_{1}v_{2}\ldots v_{n}\). Let the dragon \(C_{m} @ P_{n}\) be obtained from \(C_{m}\) and \(P_{n}\) by identifying \(u_{1}\) and \(v_{1}\). Clearly \(\left|V(C_{m} @ P_{n}))\right|+\left|E(C_{m} @ P_{n})\right|=2m+2n-2\). The proof is divided into following sixteen cases depends on the nature of \(m\) and \(n\).
Case 1. \(m\equiv 0\pmod4\) and \(n\equiv 0\pmod4\).
As in case 1 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n-2)\) as in case 1 of Theorem 1. Finally we assign the label \(3\), \(4\) to the vertices \(v_{4r-1}\) and \(v_{4r}\) respectively.
Case 2. \(m\equiv 0\pmod4\) and \(n\equiv 1\pmod4\).
As in case 1 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n)\) as in case 2 of Theorem 1. Next we relabel the vertex \(v_{4r-2}\) by \(4\).
Case 3. \(m\equiv 0\pmod4\) and \(n\equiv 2\pmod4\).
As in case 1 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n)\) as in case 3 of Theorem 1.
Case 4. \(m\equiv 0\pmod4\) and \(n\equiv 3\pmod4\).
As in case 1 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n)\) as in case 4 of Theorem 1. Then we relabel the vertex \(v_{4r-3}\) by \(4\).
Case 5. \(m\equiv 1\pmod4\) and \(n\equiv 0\pmod4\).
As in case 2 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n)\) as in case 1 of Theorem 1. Finally we relabel the vertex \(v_{4r-1}\) by \(4\).
Case 6. \(m\equiv 1\pmod4\) and \(n\equiv 1\pmod4\).
As in case 2 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n-3)\) as in case 2 of Theorem 1. Next we assign the label \(3\), \(4\), \(3\) to the vertices \(v_{4r-2}\), \(v_{4r-1}\) and \(v_{4r}\) respectively.
Case 7. \(m\equiv 1\pmod4\) and \(n\equiv 2\pmod4\).
As in case 2 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n-3)\) as in case 3 of Theorem 1. Then we assign the label \(3\), \(2\), \(3\) to the vertices \(v_{4r-2}\), \(v_{4r-1}\) and \(v_{4r}\) respectively.
Case 8. \(m\equiv 1\pmod4\) and \(n\equiv 3\pmod4\).
As in case 2 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n-3)\) as in case 4 of Theorem 1. Finally we assign the label \(2\), \(3\), \(3\) to the vertices \(v_{4r-1}\), \(v_{4r-1}\) and \(v_{4r}\) respectively.
Case 9. \(m\equiv 2\pmod4\) and \(n\equiv 0\pmod4\).
As in case 3 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n)\) as in case 1 of Theorem 1. Next we relabel the vertex \(v_{4r}\) by \(4\).
Case 10. \(m\equiv 2\pmod4\) and \(n\equiv 1\pmod4\).
As in case 3 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n)\) as in case 2 of Theorem 1. Then we relabel the vertex \(v_{4r-2}\) by \(4\).
Case 11. \(m\equiv 2\pmod4\) and \(n\equiv 2\pmod4\).
As in case 3 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n)\) as in case 3 of Theorem 1.
Case 12. \(m\equiv 2\pmod4\) and \(n\equiv 3\pmod4\).
As in case 3 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n)\) as in case 4 of Theorem 1. Finally we relabel the vertex \(v_{4r-3}\) by \(4\).
Case 13. \(m\equiv 3\pmod4\) and \(n\equiv 30\pmod4\).
As in case 4 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n)\) as in case 1 of Theorem 1. Then we relabel the vertices \(v_{r+1}\), \(v_{4r-3}\) by \(4\) and \(2\) respectively.
Case 14. \(m\equiv 3\pmod4\) and \(n\equiv 1\pmod4\).
As in case 4 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n)\) as in case 2 of Theorem 1. Next we relabel the vertex \(v_{4r-1}\) by \(4\).
Case 15. \(m\equiv 3\pmod4\) and \(n\equiv 2\pmod4\).
As in case 4 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n)\) as in case 3 of Theorem 1. Finally we relabel the vertex \(v_{4r}\) by \(4\).
Case 16. \(m\equiv 3\pmod4\) and \(n\equiv 1\pmod4\).
As in case 4 of Theorem 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq m)\) and assign the label to the vertices \(v_{i}\) \((1 \leq i \leq n)\) as in case 4 of Theorem 1. Then we relabel the vertex \(v_{4r-3}\) by \(4\). ◻
Example 1. A \(4\)-total prime cordial labeling of \(C_{12} @ P_{13}\) is given in Figure 1.

Now we investigate the \(4\)-total prime cordial labeling behavior of the graph \((C_{n}\times P_{2}) \odot K_{2}\).
Theorem 5. The corona of \(C_{n}\times P_{2}\), \((C_{n}\times P_{2}) \odot K_{2}\) is \(4\)-total prime cordial for all \(n \geq 3\).
Proof. Let \(V((C_{n}\times P_{2}) \odot K_{1})=\{u_{i}, v_{i}, w_{i}:1 \leq i \leq n\}\) and \(E((C_{n}\times P_{2}) \odot K_{1})=\{u_{1}u_{n}, v_{1}v_{n}\} \cup \{u_{i}v_{i}, v_{i}w_{i}: 1 \leq i \leq n\} \cup \{u_{i}u_{i+1}, v_{i}v_{i+1}:1 \leq i \leq n-1\}\). It is easy to verify that \(\left|V((C_{n}\times P_{2}) \odot K_{1})\right|+\left|E((C_{n}\times P_{2}) \odot K_{1})\right|=7n\).
Case 1. \(n\equiv 0\pmod4\).
Let \(n=4r\), \(r>1\) and \(r \in \mathbb{N}\). Assign the label \(4\) to the vertices \(u_{1},u_{2},\ldots,u_{r}\) and assign the label \(2\) to the vertices \(u_{r+1},u_{r+2},\ldots,u_{2r}\). Next we assign the label \(3\) to the vertices \(u_{2r+1},u_{2r+2},\ldots,u_{3r+1}\) then we assign \(1\) to the vertices \(u_{3r+2},u_{3r+3},\ldots,u_{4r-1}\). Finally we assign the label \(4\) to the vertex \(u_{4r}\). Next we consider the vertices \(v_{i}\) \((1 \leq i \leq n)\). Assign the label \(4\) to the vertices \(v_{1},v_{2},\ldots,v_{r}\) and assign the label \(2\) to the vertices \(v_{r+1},v_{r+2},\ldots,v_{2r}\) and next we assign the label \(3\) to the vertices \(v_{2r+1},v_{2r+2},\ldots,v_{3r}\). Finally we assign the label \(1\) to the vertices \(v_{3r+1},v_{3r+2},\ldots,v_{4r}\). Now we move to the vertices \(w_{i}\) \((1 \leq i \leq n)\). Assign the label \(4\) to the vertices \(w_{1},w_{2},\ldots,w_{r}\) and assign the label \(2\) to the vertices \(w_{r+1},w_{r+2},\ldots,w_{2r}\) and next we assign the label \(3\) to the vertices \(w_{2r+1},w_{2r+2},\ldots,w_{3r}\). Finally we assign the label \(1\) to the vertices \(w_{3r+1},w_{3r+2},\ldots,w_{4r}\). Clearly \(t_{f}(1)=t_{f}(2)=t_{f}(3)=t_{f}(4)=7r\).
Case 2. \(n\equiv 1\pmod4\).
Let \(n=4r+1\), \(r>1\) and \(r \in \mathbb{N}\). As in case 1, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq n-2)\), \(v_{i}\) \((1 \leq i \leq n-2)\) and \(w_{i}\) \((1 \leq i \leq n-2)\). Finally we assign the labels \(4\), \(4\), \(2\), \(1\), \(3\), \(1\) respectively to the vertices \(u_{4r}\), \(u_{4r+1}\), \(v_{4r}\), \(v_{4r+1}\), \(w_{4r}\) and \(w_{4r+1}\). Here \(t_{f}(1)=t_{f}(2)=t_{f}(4)=7r+2\) and \(t_{f}(3)=7r+1\).
Case 3. \(n\equiv 2\pmod4\).
Let \(n=4r+2\), \(r>1\) and \(r \in \mathbb{N}\). As in case 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq n-2)\), \(v_{i}\) \((1 \leq i \leq n-2)\) and \(w_{i}\) \((1 \leq i \leq n-2)\). Finally we assign the labels \(4\), \(4\), \(1\), \(3\), \(2\), \(3\) to the vertices \(u_{4r+1}\), \(u_{4r+2}\), \(v_{4r+1}\), \(v_{4r+2}\), \(w_{4r+1}\) and \(w_{4r+2}\) respectively. It is easy to verify that \(t_{f}(1)=t_{f}(2)=7r+3\) and \(t_{f}(3)=t_{f}(4)=7r+4\).
Case 4. \(n\equiv 4\pmod4\).
Let \(n=4r+3\), \(r>1\) and \(r \in \mathbb{N}\). Assign the label to the vertices \(u_{i}\) \((1 \leq i \leq n-2)\), \(v_{i}\) \((1 \leq i \leq n-2)\) and \(w_{i}\) \((1 \leq i \leq n-2)\) by case 3. Finally we assign the labels \(4\), \(4\), \(2\), \(3\), \(3\), \(3\) respectively to the vertices \(u_{4r+2}\), \(u_{4r+3}\), \(v_{4r+2}\), \(v_{4r+3}\), \(w_{4r+2}\) and \(w_{4r+3}\). Here \(t_{f}(1)=t_{f}(2)=t_{f}(3)=7r+5\) and \(t_{f}(4)=7r+6\).
Case 5. \(n=3,4,5,6,7\).
A \(4\)-total prime cordial labeling follows from Table 7.
| \(n\) | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|
| \(u_{1}\) | 4 | 4 | 4 | 4 | 4 |
| \(u_{2}\) | 2 | 2 | 2 | 4 | 4 |
| \(u_{3}\) | 3 | 3 | 3 | 2 | 2 |
| \(u_{4}\) | 4 | 2 | 2 | 2 | |
| \(u_{5}\) | 1 | 3 | 3 | ||
| \(u_{6}\) | 3 | 3 | |||
| \(u_{7}\) | 1 | ||||
| \(v_{1}\) | 4 | 4 | 4 | 4 | 4 |
| \(v_{2}\) | 2 | 2 | 2 | 4 | 4 |
| \(v_{3}\) | 3 | 3 | 3 | 2 | 2 |
| \(v_{4}\) | 3 | 3 | 3 | 2 | |
| \(v_{5}\) | 4 | 3 | 3 | ||
| \(v_{6}\) | 1 | 3 | |||
| \(v_{7}\) | 1 | ||||
| \(w_{1}\) | 4 | 4 | 4 | 4 | 4 |
| \(w_{2}\) | 3 | 2 | 2 | 2 | 4 |
| \(w_{3}\) | 3 | 3 | 3 | 2 | 2 |
| \(w_{4}\) | 1 | 3 | 3 | 3 | |
| \(w_{5}\) | 4 | 3 | 3 | ||
| \(w_{6}\) | 1 | 3 | |||
| \(w_{7}\) | 1 |
◻
Next we investigate the graph \(P_{n} \odot K_{2}\).
Theorem 6. The graph \(P_{n} \odot K_{2}\) is \(4\)-total prime cordial for all \(n\).
Proof. Let \(V(P_{n} \odot K_{2})=\{u_{i}, v_{i}, w_{i}:1 \leq i \leq n\}\) and \(E(P_{n} \odot K_{2})= \{u_{i}v_{i}, u_{i}w_{i}, v_{i}w_{i}: 1 \leq i \leq n\} \cup \{u_{i}u_{i+1}:1 \leq i \leq n-1\}\). Obviously \(\left|V(P_{n} \odot K_{2})\right|+\left|E(P_{n} \odot K_{2})\right|=7n-1\).
Case 1. \(n\equiv 0\pmod4\).
Let \(n=4r\), \(r>1\) and \(r \in \mathbb{N}\). Assign the label \(4\) to the vertices \(u_{1},u_{2},\ldots,u_{r}\) and assign the label \(2\) to the vertices \(u_{r+1},u_{r+2},\ldots,u_{2r}\). Next we assign the label \(3\) to the vertices \(u_{2r+1},u_{2r+2},\ldots,u_{3r}\). Then we assign the label \(1\) to the vertices \(u_{3r+1},u_{3r+2},\ldots,u_{4r-2}\). Finally we assign the labels \(4\), \(3\) to the vertices \(u_{4r-1}\) and \(u_{4r}\) respectively. Next we consider the vertices \(v_{i}\) \((1 \leq i \leq n)\). Assign the label \(4\) to the vertices \(v_{1},v_{2},\ldots,v_{r}\) and assign the label \(2\) to the vertices \(v_{r+1},v_{r+2},\ldots,v_{2r}\) and next we assign the label \(3\) to the vertices \(v_{2r+1},v_{2r+2},\ldots,v_{3r}\). Finally we assign the label \(1\) to the vertices \(v_{3r+1},v_{3r+2},\ldots,v_{4r}\). Now we move to the vertices \(w_{i}\) \((1 \leq i \leq n)\). Assign the label \(4\) to the vertices \(w_{1},w_{2},\ldots,w_{r}\) and assign the label \(2\) to the vertices \(w_{r+1},w_{r+2},\ldots,w_{2r}\) and next we assign the label \(3\) to the vertices \(w_{2r+1},w_{2r+2},\ldots,w_{3r}\). Finally we assign the label \(1\) to the vertices \(w_{3r+1},w_{3r+2},\ldots,w_{4r}\). Clearly \(t_{f}(1)=7r-1\) and \(t_{f}(2)=t_{f}(3)=t_{f}(4)=7r\).
Case 2. \(n\equiv 1\pmod4\).
Let \(n=4r+1\), \(r>1\) and \(r \in \mathbb{N}\). As in case 1, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq n-1)\), \(v_{i}\) \((1 \leq i \leq n-1)\) and \(w_{i}\) \((1 \leq i \leq n-1)\). Finally we assign the labels \(3\), \(4\), \(2\) respectively to the vertices \(u_{4r+1}\), \(v_{4r+1}\) and \(w_{4r+1}\). Here \(t_{f}(1)=t_{f}(4)=7r+1\) and \(t_{f}(2)=t_{f}(3)=7r+2\).
Case 3. \(n\equiv 2\pmod4\).
Let \(n=4r+2\), \(r>1\) and \(r \in \mathbb{N}\). Assign the label to the vertices \(u_{i}\) \((1 \leq i \leq n-2)\), \(v_{i}\) \((1 \leq i \leq n-2)\) and \(w_{i}\) \((1 \leq i \leq n-2)\) as in case 1. Finally we assign the labels \(4\), \(3\), \(4\), \(3\), \(2\), \(1\) to the vertices \(u_{4r+1}\), \(u_{4r+2}\), \(v_{4r+1}\), \(v_{4r+2}\), \(w_{4r+1}\) and \(w_{4r+2}\) respectively. It is easy to verify that \(t_{f}(1)=7r+4\) and \(t_{f}(2)=t_{f}(3)=t_{f}(4)=7r+3\).
Case 4. \(n\equiv 4\pmod4\).
Let \(n=4r+3\), \(r>1\) and \(r \in \mathbb{N}\). As in case 2, assign the label to the vertices \(u_{i}\) \((1 \leq i \leq n-2)\), \(v_{i}\) \((1 \leq i \leq n-2)\) and \(w_{i}\) \((1 \leq i \leq n-2)\). Finally we assign the labels \(4\), \(3\), \(4\), \(4\), \(2\), \(3\) respectively to the vertices \(u_{4r+2}\), \(u_{4r+3}\), \(v_{4r+2}\), \(v_{4r+3}\), \(w_{4r+2}\) and \(w_{4r+3}\). Here \(t_{f}(1)=t_{f}(2)=t_{f}(3)=t_{f}(4)=7r+5\).
Case 5. \(n=2,3,4,5,6,7\).
A \(4\)-total prime cordial labeling follows from Table 8.
]
| \(n\) | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|
| \(u_{1}\) | 4 | 2 | 4 | 4 | 4 | 4 |
| \(u_{2}\) | 3 | 3 | 2 | 4 | 4 | 4 |
| \(u_{3}\) | 3 | 3 | 2 | 2 | 2 | |
| \(u_{4}\) | 1 | 3 | 3 | 2 | ||
| \(u_{5}\) | 3 | 3 | 3 | |||
| \(u_{6}\) | 1 | 3 | ||||
| \(u_{7}\) | 4 | |||||
| \(v_{1}\) | 4 | 4 | 4 | 4 | 4 | 4 |
| \(v_{2}\) | 3 | 4 | 2 | 2 | 4 | 4 |
| \(v_{3}\) | 4 | 3 | 2 | 2 | 2 | |
| \(v_{4}\) | 3 | 3 | 3 | 3 | ||
| \(v_{5}\) | 1 | 3 | 3 | |||
| \(v_{6}\) | 1 | 4 | ||||
| \(v_{7}\) | 3 | |||||
| \(w_{1}\) | 2 | 4 | 4 | 4 | 4 | 4 |
| \(w_{2}\) | 1 | 3 | 2 | 2 | 2 | 2 |
| \(w_{3}\) | 2 | 3 | 3 | 2 | 2 | |
| \(w_{4}\) | 1 | 3 | 3 | 3 | ||
| \(w_{5}\) | 1 | 1 | 3 | |||
| \(w_{6}\) | 1 | 1 | ||||
| \(w_{7}\) | 1 |
◻
Next we investigate the graph \(C_{n} \odot K_{2}\).
Corollary 1. The graph \(C_{n} \odot K_{2}\) is \(4\)-total prime cordial for all \(n\).
Proof. Let \(V(P_{n} \odot K_{2})=\{u_{i}, v_{i}, w_{i}:1 \leq i \leq n\}\) and \(E(P_{n} \odot K_{2})= \{u_{1}u_{n}, v_{1}v_{n}\} \cup \{u_{i}v_{i}, u_{i}w_{i}, v_{i}w_{i}: 1 \leq i \leq n\} \cup \{u_{i}u_{i+1}:1 \leq i \leq n-1\}\). Clearly \(\left|V(C_{n} \odot K_{2})\right|+\left|E(C_{n} \odot K_{2})\right|=7n\).
Case 1. \(n\equiv 0,1,3\pmod4\).
Let \(n=4r\),\(n=4r+1\),\(n=4r+3\), \(r \in \mathbb{N}\). The vertex labelled in Theorem 6 is also a \(4\)-total prime cordial of \(C_{n}\odot K_{2}\).
Case 2. \(n\equiv 2\pmod4\).
Let \(n=4r+2\), \(r \in \mathbb{N}\). Assign the label to \(u_{i},v_{i},w_{i}\) \((1 \leq i \leq n)\) as in Theorem 6. Finally the vertex \(w_{4r+2}\) is relabelled by \(4\). Obviously this vertex labels is a \(4\)-total prime cordial of \(C_{n}\odot K_{2}\).
Case 3. \(n=2,3,4,5,6,7\).
A \(4\)-total prime cordial labeling follows from Table 9.
| \(n\) | 2 | 3 | 4 | 5 | 6 | 7 | ||
|---|---|---|---|---|---|---|---|---|
| \(u_{1}\) | 4 | 2 | 4 | 4 | 4 | 4 | ||
| \(u_{2}\) | 3 | 3 | 2 | 4 | 4 | 4 | ||
| \(u_{3}\) | – | 3 | 3 | 2 | 2 | 2 | ||
| \(u_{4}\) | – | – | 1 | 3 | 3 | 2 | ||
| \(u_{5}\) | – | – | – | 3 | 3 | 3 | ||
| \(u_{6}\) | – | – | – | – | 1 | 3 | ||
| \(u_{7}\) | – | – | – | – | – | 4 | ||
| \(v_{1}\) | 4 | 4 | 4 | 4 | 4 | 4 | ||
| \(v_{2}\) | 3 | 4 | 2 | 2 | 4 | 4 | ||
| \(v_{3}\) | – | 4 | 3 | 2 | 2 | 2 | ||
| \(v_{4}\) | – | – | 3 | 3 | 3 | 3 | ||
| \(v_{5}\) | – | – | – | 1 | 3 | 3 | ||
| \(v_{6}\) | – | – | – | – | 1 | 4 | ||
| \(v_{7}\) | – | – | – | – | – | 3 | ||
| \(w_{1}\) | 2 | 4 | 4 | 4 | 4 | 4 | ||
| \(w_{2}\) | 4 | 3 | 2 | 2 | 2 | 2 | ||
| \(w_{3}\) | – | 2 | 3 | 3 | 2 | 2 | ||
| \(w_{4}\) | – | – | 4 | 3 | 3 | 3 | ||
| \(w_{5}\) | – | – | – | 1 | 1 | 3 | ||
| \(w_{6}\) | – | – | – | – | 4 | 1 | ||
| \(w_{7}\) | – | – | – | – | – | 1 |
◻
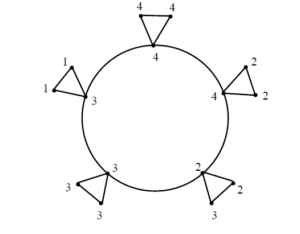
Example 2. A \(4\)-total prime cordial labeling of \(C_{5} \odot K_{2}\) is given in Figure 2.
1970-2025 CP (Manitoba, Canada) unless otherwise stated.