1. Introduction
All graphs considered in this paper are finite, simple, and
undirected. Let denote a
complete graph on vertices, a complete bipartite graph with
vertex partite sets of cardinality and , and a path on vertices. A -tree on vertices, denoted by , is a tree in which one edge is
attached to a vertex of the path
such that at least one of
the adjacent vertices of has
degree 1.
A decomposition of a graph is
a set of edge-disjoint subgraphs of such that
every edge of belongs to exactly
one , . If all the subgraphs in
the decomposition of are
isomorphic to a graph , then is said to be -decomposable. If can be decomposed into copies of and copies of , then is said to have an -multi-decomposition or an
-decomposition. The pair is called admissible if
it satisfies the necessary conditions for the existence of an -decomposition.
If has an -multi-decomposition for all
admissible pairs ,
it is said to have an -decomposition.
The necessary and sufficient condition for the existence of a -decomposition of complete graphs was
studied in [5], and for
complete bipartite graphs in [1]. The path decomposition of various graphs was
explored in [15,11]. The graph
is one of the three
non-isomorphic trees of order five, excluding paths and stars. Caterina
and Antonio [3] named
as the chair and
studied the stability number of chair-free graphs.
The -decomposition of
complete graphs was obtained by C. Huang and A. Rosa [5]. J. Paulraj Joseph and A. Samuel
Issacraj [8] referred to
as the fork and
studied its decomposition in complete bipartite graphs. S. Gomathi and
A. Tamil Elakkiya [4] defined
this graph as a -tree and
investigated its decomposition in the tensor product of complete graphs.
The -decomposition of various
graphs was further analyzed in [9,10]. The concept of multi-decomposition
was introduced by A. Abueida and M. Daven [2]. In recent years, multi-decomposition of graphs
has emerged as a prominent research area in graph theory. T.-W. Shyu
studied the multi-decomposition of complete graphs into paths with
cycles and stars [12,13]. S.
Jeevadoss and A. Muthusamy established necessary and sufficient
conditions for the multi-decomposition of complete bipartite graphs into
paths and cycles [6].
Multi-decomposition of complete bipartite graphs into paths and stars
was considered in [14].
In this paper, we establish the necessary and sufficient conditions
for the existence of a -multi-decomposition of and . To prove our results, we recall
the following theorems:
Theorem 1.1. [5] The complete graph is -decomposable if and only if .
Theorem 1.2. [1] Let and be positive
integers. The necessary conditions for a -decomposition of are listed in Table 1, and
these conditions are also sufficient.
Table 1 Necessary and sufficient conditions for a -decomposition of
|
Case
|
|
|
|
Characterization
|
|
1.
|
even
|
even
|
even
|
not both equal
|
|
2.
|
odd
|
even
|
even
|
Equalities hold when is even
|
|
3.
|
even
|
even
|
odd
|
|
|
4.
|
even
|
odd
|
even
|
|
|
5.
|
even
|
odd
|
odd
|
Decomposition impossible
|
|
6.
|
odd
|
even
|
odd
|
|
|
7.
|
odd
|
odd
|
even
|
|
|
8.
|
odd
|
odd
|
odd
|
|
Theorem 1.3. [8] The complete bipartite graph is fork-decomposable if and only
if , except for
, .
2. Multi-decomposition
of complete graphs into and
2.1. Preliminaries
Definition 2.1. [
7] For a graph
, two disjoint subsets of vertices are
called
twins if they have the same order and induce subgraphs
with the same number of edges.
Next, we introduce a new graph structure called Conjoined
Twins in the following remark.
2.2. Notations
For a subgraph of , denotes a graph where and .
denotes disjoint copies of the graph .
means
can be decomposed into and .
Let , , be the vertices of the
complete graph .
In the complete bipartite graph , the vertices of the first
partite set with vertices are
denoted by , , and the second partite
set with vertices by , .
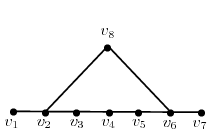
A path with vertices , , having and
as pendant vertices is denoted
by .
The graph with vertices , , is denoted by , where , , form a path of length three, and the underlined
vertices denote an edge .
Suppose we have a graph whose vertices are Conjoined
Twins as in Figure 1. We denote it by , where , , form a path of length six, and the underlined vertices
denote edges and .
2.3. Necessary condition
The following theorem gives the necessary condition for the existence
of a multi-decomposition of the complete graph into paths and -trees with 5 vertices.
Theorem 2.4. If
has a
– multi-decomposition, then
.
Proof. Proof follows from the edge divisibility
condition. 
2.4. Sufficient conditions
In this section, we show that the necessary condition obtained in
Theorem 2.4 is also sufficient for the existence of a
multi-decomposition of , () into and .
Lemma 2.5. The Complete graphs
and
have
– multi-decomposition.
Proof. , where the ’s and
are given by,
Similarly, can be written as
, where the
’s and are as follows: 
Lemma 2.6. The Complete bipartite graphs
,
and
have
– multi-decomposition.
Proof. It is clear that , where ’s are
given by,
Similarly , where
’s are identified as,
Further , the
following are the required ’s

Lemma 2.7. The graph
admits
–
decomposition when
.
Proof. The admissible pairs satisfying are
{(0,7),(1,6),(2,5),(3,4),(4,3),(5,2),
(6,1),(7,0)}.
Case 1. .
From Lemma 2.5, , which can be taken into any of the forms: using Remark 2.2.
Thus we have –
multi-decomposition for the admissible pairs
Case 2. .
Theorem 1.1 gives the required decomposition for
the admissible pair .
Hence the proof follows from Cases 1 & 2 for all admissible pairs
. 
Lemma 2.8. The graph
admits
–
decomposition if
.
Proof. The admissible pairs satisfying are
{(0,9),(1,8),(2,7),(3,6),(4,5),(5,4),
(6,3),(7,2),(8,1),(9,0)}.
Case 1. .
From Lemma 2.6, , which can be taken into any of the forms: using Remark 2.2.
Thus we have –
multi-decomposition for the admissible pairs
Case 2. .
Theorem 1.1 gives the required decomposition for
the admissible pair .
Thus, admits –
decomposition. 
Lemma 2.9. The graph
admits
–
decomposition if
.
Proof. The admissible pairs satisfying are
{(0,14),(1,13),(2,12),(3,11),(4,10),(5,9),(6,8),
(7,7),(8,6),(9,5),(10,4),(11,3),(12,2),(13,1),(14,0)}.
From Lemma 2.6, , which can be taken into any of the forms: using Remark 2.2.
Thus we have –
multi-decomposition for all the admissible pairs . 
Lemma 2.10. The graph
admits
–
decomposition if
.
Proof. The admissible pairs satisfying are
{(0,16),(1,15),(2,14),(3,13),(4,12),(5,11),(6,10),
(7,9),(8,8),(9,7),(10,6),(11,5),(12,4),(13,3),(14,2),(15,1),(16,0)}.
From Lemma 2.6 , . Then we have
– multi-decomposition for all the admissible pairs using Remark 2.2. 
Lemma 2.11. The graph
admits
–
decomposition when
.
Proof. The admissible pairs satisfying are {}.
From Lemma 2.6 , . Then we have
– multi-decomposition for all the admissible pairs using Remark 2.2. 
Theorem 2.12. (Sufficient conditions) For given
non negative integers
,
and
,
has
–
decomposition whenever
Proof. From the given (Necessary conditions) edge
divisibility condition,
we have .
Case 1: .
Let , is even. We prove this theorem using
induction on . When , the proof follows from Lemma 2.7. We
observe that for ,
Also for ,
From (1) and (2),
Assume that the theorem is true for all even . We have to prove for . From (3), we can write,
By induction hypothesis and from Lemmas 2.7, 2.8, 2.9 and 2.10 the proof
follows.
Case 2: .
Let , is even. When , the proof follows from Lemma 2.8. We
observe that for , Also for ,
From (4) and (5),
Assume that the theorem is true for all even . We have to prove for . From (6), we can write,
By induction hypothesis and from Lemmas 2.7, 2.8 and 2.11 the proof
follows. 
Theorem 2.13. (Main Theorem) For non-negative integers
,
and
,
if and only if
.
Proof. The proof follows from Theorems 2.4 and 2.12. 
3. Multi-decomposition
of complete bipartite graphs into and
3.1. Necessary conditions
In this section, we derive the necessary conditions for the existence
of multi-decomposition of ,
() into paths and
-trees with 5 vertices.
Lemma 3.1. Let
be even. If
has a
– multi-decomposition for the
admissible pair
,
then
is even.
Proof. Let , where , and . has a degree sequence . While decomposing into ’s and ’s, the two vertices of with degree 2 which are incident with
a vertex of degree 1, should be formed using the vertex set {}. has a degree sequence . Here, the vertex with degree
3 and the vertex with degree 1 which is incident with a vertex of degree
2, should be formed using the vertex set . Since each vertex in has degree , after decomposing into number of , each vertex , has degree and . Since
is even, it is clear that Therefore, partitioning the remaining edges into number of is possible only when is even. 
Lemma 3.2. Let
be odd. If
has a
– multi-decomposition for the
admissible pair
,
then
is odd.
Proof. The proof is same as Lemma 3.1 with the same
argument. Since is odd,
Hence the proof follows. 
Theorem 3.3. (Necessary conditions) If
has
–
decomposition, then
with
and
except
, even; and is odd
, odd; and is even
Proof. The proof follows from edge divisibility condition
and by Lemmas 3.1 and 3.2. 
3.2. Sufficient conditions
In the following lemmas we prove that the above necessary conditions
are also sufficient.
Proof. By Theorem 3.3, . Hence the admissible pairs are , and . By Theorem 1.2, can be decomposed into and by Theorem 1.3, can be decomposed into . Hence there exists a – multi-decomposition for the
admissible pairs and . By Lemma 3.1, it is clear that
there does not exist a –
multi-decomposition for the admissible pair . Hence the proof. 
Lemma 3.5. The graph
has
– multi-decomposition for some
of the admissible pairs (
) where
is
odd.
Proof. The admissible pairs for which the decomposition
exists are () {(3,0), (1,2)}. For , Theorem 1.2 gives the
required decomposition. For ,
we have the necessary breakdown is as follows:
The desired decomposition does not exist for the admissible pairs
and by Lemma 3.2. 
Lemma 3.6. Let
be even. If
is even in the admissible pair
, then
has a
– multi-decomposition.
Proof. Since is even,
for . we write, .
Therefore, by Lemma 3.4, for any even such that , there exists a – multi-decomposition for the
admissible pairs
with , are even. This completes the
proof. 
Lemma 3.7. Let
be odd. If
is odd, then
has
– multi-decomposition.
Proof. Since
is odd, for . we write, .
Therefore, by Lemmas 3.4 and 3.5, for any odd
such that , there exists a – multi-decomposition for the
admissible pairs
with is odd and is even. This completes the
proof. 
Lemma 3.8. The graph
admits
–
decomposition whenever
.
Proof. Case 1: (3,0).
Theorem 1.2 gives required 3’s.
Case 2: (2,1).
Case 3: (1,2).
Case 4: (0,3).
Theorem 1.3 gives the required decomposition. 
Lemma 3.9. The graph
admits
–
decomposition whenever
.
Proof. Case 1: is even i.e., {(4,0), (2,2), (0,4)}.
Since , Theorems
1.2 and 1.3 give the
required decomposition.
Case 2:
is odd.
Subcase 1: (3,1).
Subcase 2: (1,3). 
Lemma 3.10. The graphs
and
admits
–
decomposition whenever
and
respectively.
Proof. We can write , . Then the proof follows from Lemmas 3.4 and 3.8. 
Lemma 3.11. The graph
admits
–
decomposition whenever
.
Proof. We can write . Since , the proof follows
from Lemmas 3.5 and 3.10. 
Lemma 3.12. If
,
,
then
can be decomposed
into admissible pairs of
and
.
Proof. Let
for and .
If , .
For , .
When , .
When , .
Then the proof follows from Lemmas 3.8, 3.9, 3.10 and by
mathematical induction on , . 
Lemma 3.13. If
be odd, then
can be decomposed into admissible pairs of
and
.
Proof. Since , are odd,
and for and we write,
.
Then the proof follows from Lemmas 3.9, 3.10, 3.11 and by
mathematical induction on ,
. 
Theorem 3.14. (Sufficient Conditions) If
and
satisfy the necessary condition
given in Theorem 3.3, then
has
–
decomposition.
Proof. Case 1: or , w.l.o.g,
let for .
Subcase 1.1. .
Lemma 3.6 gives the required
decomposition.
Subcase 1.2. .
Lemma 3.12 gives the required decomposition.
Case 2: and , i.e., , for , .
Subcase 2.1. When one of them is , w.l.o.g, let .
When is even, this falls in
Subcase 1.1. If is odd,
Lemma 3.7 gives the required
decomposition.
Subcase 2.2. .
When one of and or both of them are even, then the
proof follows from Subcase 1.2. If both of them are odd, Lemma 3.13 gives
the required decomposition. 
Theorem 3.15. (Main Theorem) There exists
– decomposition of
if and only if any one of the
following holds:
is even, and is even.
is odd, and is odd.
and .
and ; where are odd.
Proof. Proof follows from Theorems 3.3 and 3.14. 
4. Conclusion
In this paper, it is proved that the necessary and sufficient
condition for the existence of the – decomposition of the complete graph
() is . Also we have obtained the necessary and sufficient
conditions for the – decomposition of the complete bipartite graph (, ) as whenever
, even; then is even.
, odd; then is odd.